Car on a Banked Circular Road: Angle to Make the Loads Equal

[Master1022]

Homework Statement

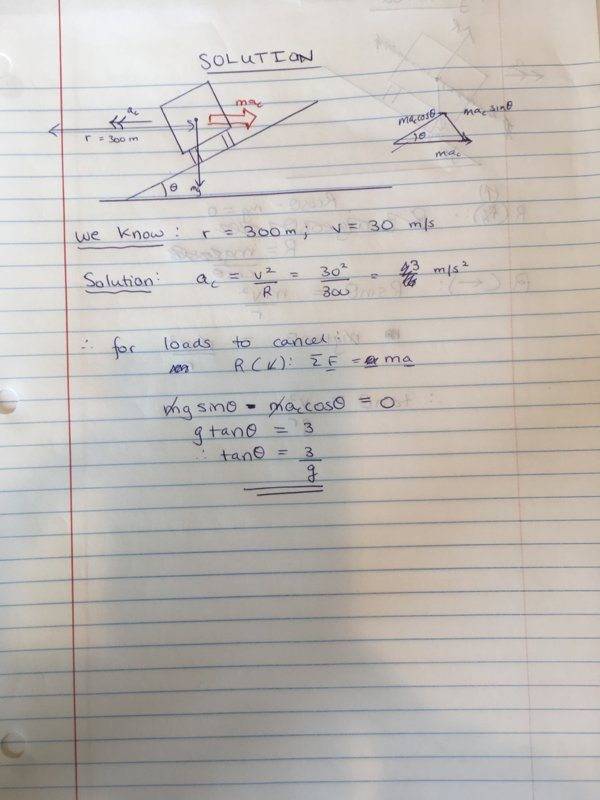
A car travels around a banked curve at a steady speed of 30 m/s. Calculate the angle, theta, of the bank required to make the load carried by the wheels on each side of the car the same.

Relevant Equations

In the attached image

Hi, I was wondering whether I could get some help on this problem. I think my questions arise from a misunderstanding. I have attached an image of my working and an image of the solution copied out (that is all it says, doesn't have much explanation). I know the working I attached was for a different type of problem, but the answers come out in the same form which leads me to wonder whether the questions are the same but under separate guises.

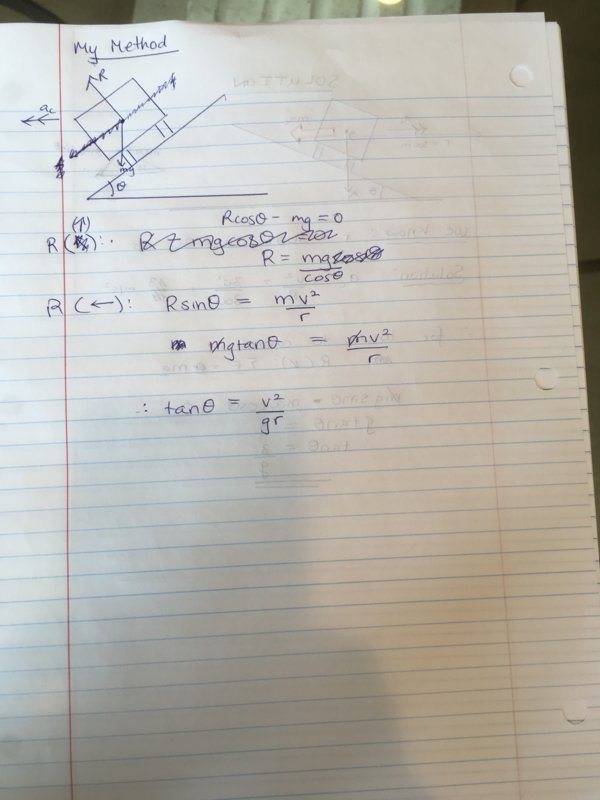I think my questions boil down to the following:
1. What are the conditions for the loads to be the same and what are the reasons behind it?
- from the solution, it seems as it just has to do with acceleration balance parallel to the slope. However, when thinking about the reaction force balance, I would normally think about moment equilibrium. I would take moments about the centre of mass of the car for two separate reaction forces, N1 and N2 to get a relation there, and then resolve horizontally and vertically.

2. When should I include the 'fictitious' ma forces?
- when I learned mechanics, we were advised to avoid doing this altogether.

3. Is there a better/ another method of solving this problem?

Thank you in advance.

(P.S. Does anyone know how I can get the images to just be a link to them, rather than having the whole image in the picture)

 

[kuruman]

You have a "Solution" and you have a "My method". Where is it said in either one that the work shown will lead to the load on the inner and outer wheels be the same? In other words, if $R_1$ and $R_2$ are the loads on the inner and outer sets of wheels, how is it guaranteed that $R_1=R_2$?

 

[Master1022]

You have a "Solution" and you have a "My method". Where is it said in either one that the work shown will lead to the load on the inner and outer wheels be the same? In other words, if $R_1$ and $R_2$ are the loads on the inner and outer sets of wheels, how is it guaranteed that $R_1=R_2$?

Thank you for your response. The 'my solution' was really supposed to be the solution to the other standard 'car on a banked road question' and was just showing that the answers came out as being of the same form, thus leading me to wonder whether the original problem could be solved another way.

With regards to the actual condition, that is where I am confused. It is an old past paper, so the solutions are handwritten and all it says is 'for side loads to cancel, then:' and the working is as I have copied out.

 

[kuruman]

I am wondering if there are two separate problems here. The wheels are separated by a distance, so the outer wheels describe a larger circle than the inner wheels. In that case, the question about equalizing the loads would make sense if the wheelbase is provided in the statement of the problem which it isn't. The solution you provide is appropriate to a "point" car, i.e. all points of the car are at the same distance from the center of the circle. However if that's the case, there is no issue about equalizing the loads since there are no inner and outer wheels by assumption. Do you see what I'm saying about two separate problems?

 

[haruspex]

The wheels are separated by a distance, so the outer wheels describe a larger circle than the inner wheels. In that case, the question about equalizing the loads would make sense if the wheelbase is provided in the statement of the problem which it isn't.

It's easiest to treat it in the frame of the vehicle. The centrifugal force and the weight of the vehicle exert opposing torques about a point midway between the points where the wheels contact the road.

 

[kuruman]

It's easiest to treat it in the frame of the vehicle. The centrifugal force and the weight of the vehicle exert opposing torques about a point midway between the points where the wheels contact the road.

That is true, but to calculate the torques about the point you mentioned, isn't it also true that you will need the height $H$ of the CM above the road surface and the distance $2L$ between the left and right tires? (Maybe the ratio $H/L$ will suffice, I am not sure.)

 

[Master1022]

I am wondering if there are two separate problems here. The wheels are separated by a distance, so the outer wheels describe a larger circle than the inner wheels. In that case, the question about equalizing the loads would make sense if the wheelbase is provided in the statement of the problem which it isn't. The solution you provide is appropriate to a "point" car, i.e. all points of the car are at the same distance from the center of the circle. However if that's the case, there is no issue about equalizing the loads since there are no inner and outer wheels by assumption. Do you see what I'm saying about two separate problems?

Thank you for your response. Given the lack of data, perhaps this question wants us to treat the car as a point mass, but phrased the question in such a way as to confuse us? I think if they wanted us to use moments, a ratio of the dimensions would need to be present in the answer.

 

[jbriggs444]

That is true, but to calculate the torques about the point you mentioned, isn't it also true that you will need the height $H$ of the CM above the road surface and the distance $2L$ between the left and right tires? (Maybe the ratio $H/L$ will suffice, I am not sure.)

If there were an asymmetric load which we were trying to equalize then the H/L ratio would enter in. For a symmetric load (i.e. centered midway between the right and left wheels), all that matters is that it is balanced.

I assume that we are dealing with a vehicle which is narrow compared to the radius of the curve so that we need not concern ourselves with the fact that the centrifugal [pseudo-]force field is non-uniform.

 

[kuruman]

If there were an asymmetric load which we were trying to equalize then the H/L ratio would enter in. For a symmetric load (i.e. centered midway between the right and left wheels), all that matters is that it is balanced.

Of course. I see it now. Assuming a symmetric load, in the non-inertial frame of the car the resultant of the horizontal centrifugal force and the vertical weight must be made perpendicular to the inclined road surface. This condition is independent of H and L.

 

[Master1022]

Of course. I see it now. Assuming a symmetric load, in the non-inertial frame of the car the resultant of the horizontal centrifugal force and the vertical weight must be made perpendicular to the inclined road surface. This condition is independent of H and L.

I am sorry to ask, but would you be able to explain again in another way as I am still struggling to understand. Thanks

 

[haruspex]

I am sorry to ask, but would you be able to explain again in another way as I am still struggling to understand. Thanks

Are you comfortable with non-inertial frames, or do you need it explained in the ground frame?

 

[kuruman]

Picture A. Imagine driving a car and there is a book with a slippery cover on the back seat. If you turn the wheel to the left so that the car goes around in a circle, the book will slide to the right, away from the center of the circle. You, being at rest with respect to the car, deduce that a force is acting on the book in a direction away from the center and call this the centrifugal force.

Picture B. Imagine the car at rest on an incline so that the left side is lower than the right side. If you place the same book on the back seat it will slide to the left because of gravity.

Picture A + B. Imagine the car on an incline turning to the left in a circle at the same time. The book on the back seat will have a downhill component of the force of gravity and an uphill component of the centrifugal force acting on it. If the car is moving at the right speed, the centrifugal component can be made to cancel the component of gravity. In that case, the net force on the book will only have a component perpendicular to the seat and the book will not slide. The same would apply for any other part of the car which means that the situation (as far as parallel forces are concerned) is as if the car were at rest on level ground with only gravity acting on it. Therefore if the load is symmetric it will stay symmetric.

I don't know if you still don't understand the argument, but I cannot explain it any better.

On edit: After seeing #11 by @haruspex, I should add that this explanation is in the non-inertial frame, i,e, the frame in which the driver is at rest.

 

[Master1022]

If the car is moving at the right speed, the centrifugal component can be made to cancel the component of gravity. In that case, the net force on the book will only have a component perpendicular to the seat and the book will not slide. The same would apply for any other part of the car which means that the situation (as far as parallel forces are concerned) is as if the car were at rest on level ground with only gravity acting on it. Therefore if the load is symmetric it will stay symmetric.

Thank you for your response. I understood this much, I was struggling to connect the dots. It makes more sense now. So we are doing this from the reference point of inside the car, which is why we include the ma force on it? If we were to do is as a bystander, then we don't include the ma force?

 

[Master1022]

Are you comfortable with non-inertial frames, or do you need it explained in the ground frame?

Thank you for your response. Terminology wise, not really, but I may have seen the concept elsewhere. Am I correct in thinking that this is the reference frame where you are accelerating along with the object, and thus relative to you it experiences no acceleration (thus leading to the rise of the 'ma' forces)?

So for the symmetrical loading, I just want to check if my logic is correct when explaining in the two different frames?
1. Inertial - the component of the weight down the slope can need only cause an acceleration that is equal to the centripetal acceleration resolved parallel to the slope
2. Non inertial - no net acceleration, so the mg\sin(\theta) must cancel out the centrifugal force parallel to the slope.

Thanks in advance

 

[haruspex]

Thank you for your response. Terminology wise, not really, but I may have seen the concept elsewhere. Am I correct in thinking that this is the reference frame where you are accelerating along with the object, and thus relative to you it experiences no acceleration (thus leading to the rise of the 'ma' forces)?

So for the symmetrical loading, I just want to check if my logic is correct when explaining in the two different frames?
1. Inertial - the component of the weight down the slope can need only cause an acceleration that is equal to the centripetal acceleration resolved parallel to the slope
2. Non inertial - no net acceleration, so the mg\sin(\theta) must cancel out the centrifugal force parallel to the slope.

Thanks in advance

Yes, that’s all correct, but for this problem we also need to think about torque.

In the frame of reference of the vehicle, we have the centrifugal force and the weight acting at the mass centre, and the normal forces and lateral friction forces acting at the wheels.
If we take moments about the point on the road midway between the wheels, the frictional forces have no moment. If the wheels are equally loaded then the torques from the normal forces on the wheels cancel.
This leaves the weight and the centrifugal force, so their torques must cancel too. I.e. their resultant must pass through the axis about which we are taking moments. The rest is simple gemetry.

 

[Master1022]

Yes, that’s all correct, but for this problem we also need to think about torque.

In the frame of reference of the vehicle, we have the centrifugal force and the weight acting at the mass centre, and the normal forces and lateral friction forces acting at the wheels.
If we take moments about the point on the road midway between the wheels, the frictional forces have no moment. If the wheels are equally loaded then the torques from the normal forces on the wheels cancel.
This leaves the weight and the centrifugal force, so their torques must cancel too. I.e. their resultant must pass through the axis about which we are taking moments. The rest is simple gemetry.

Sure, this makes much more sense now. Thank you both for taking the time to explain this to me.