Cauchy's Formula for Stress tensor

[Trying2Learn]

TL;DR Summary

Prove the area projection

Hello

I am reviewing the proof of Cauchy's formula for the stress tensor and surface traction.

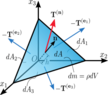
Without exception, every book I look at gets to the critical point of USING the projection of a triangle onto one of the three orthogonal planes.

However, I have never seen this proven.

1. I have read hand-waving arguments about rotating the triangle and observing the result.
2. I have read that it is possible to prove it easily with differential forms.
3. I have read statements that one begins with the area of a triangle as half the cross product of the two vectors emanating from a common vertex.
4. etc.

But I have never seen a simple proof.

Can someone help? Why do textbooks gloss over this? I have the projection is correct, but I have never seen a proof.

For example, in the attached figure, can someone point me to a GEOMETRIC (not algebraic with differential forms) proof that the area of the BLUE triangle, times, say, the direction cosine between its normal, and, say, the x1 axis vector, gives the area of the back wall.

I mean, long ago, Cauchy used it. So it cannot be that difficult, but I cannot prove it to myself, and every textbook I read in mechanical engineering (elasticity theory) glosses over this point. Why? I feel so stupid.

 

[Chestermiller]

You are asking why the projection of an area onto a surface is equal to the area times the dot product of the normal to the area with the normal to the surface, correct?

 

[FactChecker]

The geometric / mathematical proof of such projections (and many other geometric results) is treated well in the subject of geometric algebra. There is a significant learning curve, but it is an approach that gives you a methodical intuition into many geometric operations in $\mathbb{R}^n$ such as projections, reflections, and rotations.

 

[Trying2Learn]

You are asking why the projection of an area onto a surface is equal to the area times the dot product of the normal to the area with the normal to the surface, correct?

Yes... Exactly that.

I know that it can be done with differential forms (and it is motivating me to learn it).

But when I read the Cauchy Stress results, every single textbook glosses over this.

I have attempted to work it out with trig rules and cross products and determinants but I get stuck (mostly because I start doubting myself).

When I read the textbooks on elasticity, every book states this projection formula but not a single author has the human decency to say "the proof is beyond the scope of this book."

As FactChecker comments above, there is a steep learning curve. Maybe so, but it sure would be nice for the engineering authors to admit this. As of now, considering the speed and dismissive tone they take, I assume that the proof should be easy.

(Yes, I can do it for an inclined and projected rectangle, and I can do the projection for a line, but I cannot seem to prove this result, easily and I am flummoxed.)

 

[Trying2Learn]

The geometric / mathematical proof of such projections (and many other geometric results) is treated well in the subject of geometric algebra. There is a significant learning curve, but it is an approach that gives you a methodical intuition into many geometric operations in $\mathbb{R}^n$ such as projections, reflections, and rotations.

My response to Chestmiller also is to you. Are you saying that this projection proof is NOT simple and obvious?

I can live with that and begin the process of studying the geometry, as long as I know in advance.

The elasticity books make me feel like I am an idiot because I do not see this result as easily as they SEEM to suggest.

 

[Chestermiller]

If you can do it for a rectangle, then, to me that’s all it takes. Imagine a plane containing the two normals. Take rectangles within the original area perpendicular to that plane. Then add them up.

 

[Trying2Learn]

If you can do it for a rectangle, then, to me that’s all it takes. Imagine a plane containing the two normals. Take rectangles within the original area perpendicular to that plane. Then add them up.

Every one says that, but then they wave their hands and say "imagine." And I am beginning to give up and accept it, blindly.

BTW: I CAN do it if the rectangle is inclined to the plane, equally from both edges.
(Like lifting a panel off the floor with the edge of the panel against the corner. How do you do it for ANY inclined TRIANGLE). IN other words... if the rectangle is in the XY plane, with its LONGER edge against the x axis, and I lift it up, so that the edge of the rectangle remains along the x axis, I can do it.

But I cannot do it for any old orientation. And I cannot see how it would apply to the Cauchy tetrahedron. Because I no longer believe it is as easy as the engineering authors say it is. I certainly believe the result, as it makes sense, but I just do not think it is a one-line, obvious.

 

[Chestermiller]

Every one says that, but then they wave their hands and say "imagine." And I am beginning to give up and accept it, blindly.

BTW: I CAN do it if the rectangle is inclined to the plane, equally from both edges.
(Like lifting a panel off the floor with the edge of the panel against the corner. How do you do it for ANY inclined TRIANGLE). IN other words... if the rectangle is in the XY plane, with its LONGER edge against the x axis, and I lift it up, so that the edge of the rectangle remains along the x axis, I can do it.

But I cannot do it for any old orientation. And I cannot see how it would apply to the Cauchy tetrahedron. Because I no longer believe it is as easy as the engineering authors say it is. I certainly believe the result, as it makes sense, but I just do not think it is a one-line, obvious.

I really don't see what your problem is. Just use rectangles of differential thickness with long edges that run from one edge of the triangle to the other (not necessarily aligned with the coordinate directions). Just integrate over all the rectangles.

 

[Trying2Learn]

I really don't see what your problem is. Just use rectangles of differential thickness with long edges that run from one edge of the triangle to the other (not necessarily aligned with the coordinate directions). Just integrate over all the rectangles.

Then I have not made myself clear. Please let me try again.

Even on this website, there have been people asking about this (I just did a search).

So I know I am not the only one.

The issue is not the proof -- I am sure that with sufficient patience (I have little right now), I can work this out myself. And I intuitively know (from the little I have read about differential forms) that that is an elegant way to prove the projection rule.

However, the issue for me is that every single elasticity book I have read, gets to the Cauchy formula and just states the direction cosines for the projection, as if it were obvious. That is the issue for me. It is not obvious.

And as I read the other posts here on this issue (or search the internet), every attempt to explain it begins with "Imagine... blah blah blah." I don't want to imagine.

At this point I am only hoping someone can say to me (just for the sake of clarity): "The elasticity books do a major disservice, because this is not a trivial matter."

I hope that makes sense.

 

[Chestermiller]

Take the edge where the skew plane intersects the y-z plane. Draw a series of parallel lines in the skew plane parallel to that edge, and intersecting the other two edges. Form rectangles of differential height within the skew plane using these parallel lines. The width of the projection of each of these rectangles onto the y-z plane is equal to the width in the skew plane times the cosine of the angle between skew plane and the y-z plane. This angle is the same as that between the normal to the skew plane and the normal to the y-z plane. So the cosine of the angle between the planes is equal to the direction cosine in the x-diirection of the normal to the skew plane.

 

[FactChecker]

My response to Chestmiller also is to you. Are you saying that this projection proof is NOT simple and obvious?

I can live with that and begin the process of studying the geometry, as long as I know in advance.

The elasticity books make me feel like I am an idiot because I do not see this result as easily as they SEEM to suggest.

I'm sorry, I didn't really think about simple and direct proofs. There probably are some but I may not be sharp enough to come up with one. I will leave this to others for now and think about it.

 

[FactChecker]

How do you do it for ANY inclined TRIANGLE). IN other words... if the rectangle is in the XY plane, with its LONGER edge against the x axis, and I lift it up, so that the edge of the rectangle remains along the x axis, I can do it.

A triangle is just half of a rectangle and the case of a rectangle in any orientation should be able to be done by a sequence of steps to orient it in the most easily proven ways.

 

[Trying2Learn]

Hello all

(I was away for a week and am now returning to this.)

In my spare time, I worked out what I wanted to. I do not know why I was unable to express what I needed to see, but I eventually did, and worked it out myself. (I also don't know why it flummoxed me--it is not that difficult). It is attached (for those who want to see what I meant, and for those who are also interested).

Thank you for your patience, anyway.