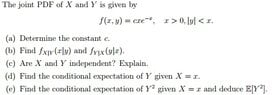- #1
nacho-man
- 171
- 0
Please refer to the attached image.
I can't quite get the bounds for question a) right. it's so confusing. Would it be wise to split the double integral into two parts? I guess that's usually favourable with when dealing with absolute values. But the bounds are still confusing me.
would it be easier to integrate with respect to x first, or w.r.t y?For x, it would just be 0 to infinity.
for y it would be -infinity to x ?whilst splitting the integral with respect to x? is this correct.
Actually i'll have a crack at it now. some as i make these question threads i tend to find a solution/or at least get closer to it.
Still, help/correction is very much appreciated.
Thanks!
edit: first attempt i did was
edit 2: This also did not workedit 3: SOLVED PART A)
i used the bounds -x<y<x and 0<x<infinity
and obtained c= 1/2
edit 4: SOLVED PART B)
I got fy|x(x,y) =
and fx|y(x,y) =
edit 5: I'm having trouble with part d
E[Y|X=e] =
I can't quite get the bounds for question a) right. it's so confusing. Would it be wise to split the double integral into two parts? I guess that's usually favourable with when dealing with absolute values. But the bounds are still confusing me.
would it be easier to integrate with respect to x first, or w.r.t y?For x, it would just be 0 to infinity.
for y it would be -infinity to x ?
Actually i'll have a crack at it now. some as i make these question threads i tend to find a solution/or at least get closer to it.
Still, help/correction is very much appreciated.
Thanks!
edit: first attempt i did was
edit 2: This also did not workedit 3: SOLVED PART A)
i used the bounds -x<y<x and 0<x<infinity
and obtained c= 1/2
edit 4: SOLVED PART B)
I got fy|x(x,y) =
and fx|y(x,y) =
edit 5: I'm having trouble with part d
E[Y|X=e] =
Attachments
Last edited: