barryj
- 856
- 51
- Homework Statement
- Would a person’s weight change if the Earth stopped rotating?
- Relevant Equations
- F = GM1M2/R^2
A = w^2R
I am confused. See my diagram below. With the Earth rotating, I think the scale would read the force of gravity or 448.4 N. If the Earth were not rotating, would the scale read less or more due to the effect of centripetal force? I tend to think more by an amount of 1.78 N. Is this correct?
If there were no Earth, and the 50 kg person was rotating around a point the centripetal force should be 1.78N. So how does the centripetal force add or subtract from gravity.
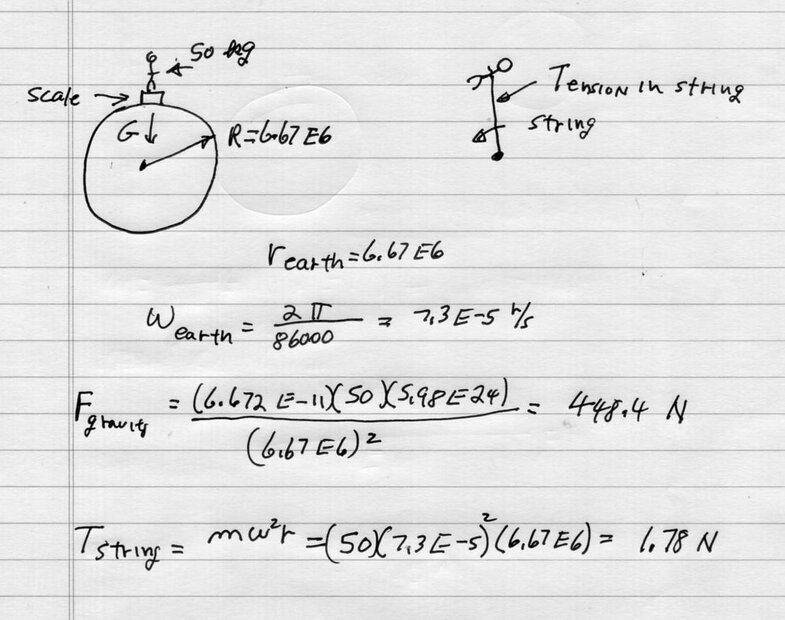
If there were no Earth, and the 50 kg person was rotating around a point the centripetal force should be 1.78N. So how does the centripetal force add or subtract from gravity.