Change of entropy in the Universe in a thermodynamic cycle

[lorenz0]

Homework Statement

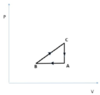
A thermodynamic cycle has $n=40 mol$ of ideal diatomic gas, initially at (A) with $P_A=1.0*10^5 Pa$ and $V_A=4m^3$, then goes to (B) via an isobaric compression such that $V_B=\frac{V_A}{2}$, followed by an expansion described by $P=k\cdot V$ such that $V_C=V_A=4m^3$ and followed by an isocorci transformation that brings the gas back to state (A).
(a) Find the work done by the gas in one cycle, the heat from the gas to the environment in a cycle and the efficiency of the engine;
(b) Suppose now the engine can exchange heat with two sources: it gives off heat to the environment at temperature $T_{env}=300K$ and absorbs heat from a source at $T_{s}=6000K.$ Find out if the machine is reversible or irreversible and determine the variation of the entropy of the universe in one cycle.

Relevant Equations

$W=-p\Delta V$, $Q=nC_V \Delta T$, $\eta=1-\frac{T_c}{T_h}$, $PV=nRT$

(a) We first find that: $T_A=\frac{P_A V_A}{nR}=\frac{1\cdot 10^5 \cdot 4}{40\cdot 8.314}K\approx 1202.7904 K$, $\frac{T_B}{T_A}=\frac{\frac{P_B V_B}{nR}}{\frac{P_A V_A}{nR}}=\frac{P_B V_B}{P_A V_A}=\frac{P_A \frac{V_A}{2}}{P_A V_A}=\frac{1}{2}$, $\frac{T_C}{T_B}=\frac{P_C V_C}{nR}\frac{nR}{P_B V_B}=\frac{kV_C V_C}{kV_B V_B}=\frac{kV_A V_A}{k \frac{V_A}{2}\frac{V_A}{2}}=4$ so $T_C=4T_B=4\frac{T_A}{2}=2T_A$. Also $P_B=\frac{nRT_B}{V_B}=\frac{nR\frac{T_A}{2}}{\frac{V_A}{2}}=\frac{nRT_A}{V_A}=P_A=\frac{40\cdot 8.314\cdot 1202.7904}{4}Pa\approx 100,000 Pa$, $P_C=\frac{nRT_C}{V_C}=\frac{nR\cdot 2T_A}{V_A}=\frac{40\cdot 8.314\cdot 2\cdot 1202.7904}{4}Pa\approx 200,000 Pa$ so $k=\frac{P_C-P_B}{V_C-V_B}=\frac{200,000-100,000}{4-2}\frac{Pa}{m^3}=50,000 \frac{Pa}{m^3}.$ We also note that, the gas being diatomic, it is $C_P=\frac{7}{2}R$ and $C_V=\frac{5}{2}R.$

#A\to B:## $W_{A\to B}=-P_A(V_B-V_A)=200,000\ Joule$, Q_{A\to B}=nC_P(T_B-T_A)=-700,000\ Joule.##
$B\to C:$ $W_{B\to C}=\int_{V_B}^{V_C}P dV=\int_{V_B}^{V_C}kV dV=\frac{k}{2}(V_C^2-V_B^2)=300,000\ Joule.$
$Q_{B\to C}=\Delta U-W_{B\to C}=nC_V(T_C-T_B)-W_{B\to C}=1,200,000\ Joule.$

$C\to A:$ $W_{C\to A}=0\ Joule$, $Q_{C\to A}=nC_V(T_A-T_C)=-1,000,000\ Joule.$

So the total work done is $W_{TOT}=(200,000+300,000)\ Joule=500,000\ Joule$, the heat given to the environment $Q_{g}=(700,000+1,000,000)\ Joule=1,700,000\ Joule$ and the efficiency is $\eta=\frac{W_{done}}{Q_{absorbed}}=\frac{200,000+300,000}{1,200,000}=\frac{5}{24}.$

(b) With the given sources temperatures we have that $\eta_{ideal}=1-\frac{T_{cold}}{T_{hot}}=1-\frac{300}{6000}=\frac{19}{20}>\eta$ so the engine is not ideal (irreversible).My question now is: how do I find the change in entropy of the universe? I know how to find the entropy change in each step of the cycle by applying $\Delta S=nC_V\ln(\frac{T_f}{T_i})+nR\ln(\frac{V_f}{V_i})$ but how do I go from that to finding the entropy change of the Universe? Thanks.

 

[TSny]

Your calculations basically looks good. But there are some sign errors when calculating the work. It's important to keep in mind whether you're calculating the work done by the gas or the work done $on$ the gas.

Relevant Equations:: $W=-p\Delta V$, $Q=nC_V \Delta T$, $\eta=1-\frac{T_c}{T_h}$, $PV=nRT$

Regarding the first equation, does it represent the work done by the gas or the work done $on$ the gas?

(a) We first find that: $T_A=\frac{P_A V_A}{nR}=\frac{1\cdot 10^5 \cdot 4}{40\cdot 8.314}K\approx 1202.7904 K$, $\frac{T_B}{T_A}=\frac{\frac{P_B V_B}{nR}}{\frac{P_A V_A}{nR}}=\frac{P_B V_B}{P_A V_A}=\frac{P_A \frac{V_A}{2}}{P_A V_A}=\frac{1}{2}$, $\frac{T_C}{T_B}=\frac{P_C V_C}{nR}\frac{nR}{P_B V_B}=\frac{kV_C V_C}{kV_B V_B}=\frac{kV_A V_A}{k \frac{V_A}{2}\frac{V_A}{2}}=4$ so $T_C=4T_B=4\frac{T_A}{2}=2T_A$. Also $P_B=\frac{nRT_B}{V_B}=\frac{nR\frac{T_A}{2}}{\frac{V_A}{2}}=\frac{nRT_A}{V_A}=P_A=\frac{40\cdot 8.314\cdot 1202.7904}{4}Pa\approx 100,000 Pa$, $P_C=\frac{nRT_C}{V_C}=\frac{nR\cdot 2T_A}{V_A}=\frac{40\cdot 8.314\cdot 2\cdot 1202.7904}{4}Pa\approx 200,000 Pa$ so $k=\frac{P_C-P_B}{V_C-V_B}=\frac{200,000-100,000}{4-2}\frac{Pa}{m^3}=50,000 \frac{Pa}{m^3}.$ We also note that, the gas being diatomic, it is $C_P=\frac{7}{2}R$ and $C_V=\frac{5}{2}R.$

These look good. I don't know why you bothered to calculate $P_B$. You know that A→B is isobaric and you are given $P_A$.

Also, it's not necessary to find the temperatures. Later, when you want to calculate, say, $nC_P(T_B-T_A)$ you can write this as $n\frac{7}{2}R(T_B-T_A) = \frac{7}{2}(nRT_B-nRT_A) = \frac{7}{2}(P_BV_B-P_AV_A$).

So, we don't actually need the temperatures. But, it's certainly OK to find the temperatures if you want.

$A\to B:$ $W_{A\to B}=-P_A(V_B-V_A)=200,000\ Joule$

Is this the work done by the gas or the work done on the gas?

$B\to C:$ $W_{B\to C}=\int_{V_B}^{V_C}P dV=\int_{V_B}^{V_C}kV dV=\frac{k}{2}(V_C^2-V_B^2)=300,000\ Joule.$

Is this the work done by the gas or on the gas?

$Q_{B\to C}=\Delta U-W_{B\to C}=nC_V(T_C-T_B)-W_{B\to C}=1,200,000\ Joule.$

Does the symbol $W_{B\to C}$ here represent the work done on the gas or the work done by the gas?

$C\to A:$ $W_{C\to A}=0\ Joule$, $Q_{C\to A}=nC_V(T_A-T_C)=-1,000,000\ Joule.$

OK

So the total work done is $W_{TOT}=(200,000+300,000)\ Joule=500,000\ Joule$, the heat given to the environment $Q_{g}=(700,000+1,000,000)\ Joule=1,700,000\ Joule$ and the efficiency is $\eta=\frac{W_{done}}{Q_{absorbed}}=\frac{200,000+300,000}{1,200,000}=\frac{5}{24}.$

These are not the correct results due to sign errors in calculating the work. As a check, you should find that the total heat added to the gas during a cycle equals the total work done by the gas during the cycle. This follows from the first law and the fact that the internal energy is a state variable. So, $\Delta U_{\rm cycle} = 0$.

My question now is: how do I find the change in entropy of the universe? I know how to find the entropy change in each step of the cycle by applying $\Delta S=nC_V\ln(\frac{T_f}{T_i})+nR\ln(\frac{V_f}{V_i})$ but how do I go from that to finding the entropy change of the Universe? Thanks.

The change in entropy of the universe is $$\Delta S_{\rm univ} = \Delta S_{\rm hot \, reservoir} + \Delta S_{\rm cold \, reservoir} + \Delta S_{\rm gas}$$
Can you find each term on the right?

 

[Delta2]

@TSny, the problem statement doesn't make reference to hot and cold reservoir, I get it that the first is the source and the second is the environment?

 

[TSny]

@TSny, the problem statement doesn't make reference to hot and cold reservoir, I get it that the first is the source and the second is the environment?

I interpret part (b) as saying that the engine goes through the same cycle as part (a). But any heat that is transferred to the gas comes from a hot reservoir at 6000 K and any heat that leaves the gas goes to a cold reservoir at 300 K. Maybe that's not the intended interpretation.

 

[Delta2]

I see now , thanks.

 

[lorenz0]

Your calculations basically looks good. But there are some sign errors when calculating the work. It's important to keep in mind whether you're calculating the work done by the gas or the work done $on$ the gas.Regarding the first equation, does it represent the work done by the gas or the work done $on$ the gas?These look good. I don't know why you bothered to calculate $P_B$. You know that A→B is isobaric and you are given $P_A$.

Also, it's not necessary to find the temperatures. Later, when you want to calculate, say, $nC_P(T_B-T_A)$ you can write this as $n\frac{7}{2}R(T_B-T_A) = \frac{7}{2}(nRT_B-nRT_A) = \frac{7}{2}(P_BV_B-P_AV_A$).

So, we don't actually need the temperatures. But, it's certainly OK to find the temperatures if you want.Is this the work done by the gas or the work done on the gas?Is this the work done by the gas or on the gas?Does the symbol $W_{B\to C}$ here represent the work done on the gas or the work done by the gas?OKThese are not the correct results due to sign errors in calculating the work. As a check, you should find that the total heat added to the gas during a cycle equals the total work done by the gas during the cycle. This follows from the first law and the fact that the internal energy is a state variable. So, $\Delta U_{\rm cycle} = 0$.

The change in entropy of the universe is $$\Delta S_{\rm univ} = \Delta S_{\rm hot \, reservoir} + \Delta S_{\rm cold \, reservoir} + \Delta S_{\rm gas}$$
Can you find each term on the right?

Thank you very much for your detailed answer. The work is to be intended as the work done ON the gas, so $W_{B\to C}$ should have been $W_{B\to C}=-300,000\ Joule$ and now the total work done by the gas during a cycle is equal to the heat added to the gas during a cycle.

Regarding the entropy change of the universe, the entropy change of the gas during one cycle should be $0$, since entropy is a state variable. So $\Delta S_{universe}=-\frac{Q_{B\to C}}{T_{hot}}+\frac{|Q_{A\to B}|+|Q_{C\to A}|}{T_{cold}}=-\frac{1800000 J}{6000K}+\frac{(700000+1000000)J}{300K}\approx 5366.7 J/K$. Is this correct? Thanks

 

[TSny]

Yes, that all looks good. At least that's what I get also.

 

[lorenz0]

Yes, that all looks good. At least that's what I get also.

But now, the efficiency should be $\eta=\frac{W_{done}}{Q_{absorbed}}=\frac{300,000}{1800000}=\frac{1}{6}<\frac{19}{20}=1-\frac{T_{cold}}{T_{hot}}=\eta_{Carnot}$ so it is still not a reversible cycle, right?

 

[TSny]

But now, the efficiency should be $\eta=\frac{W_{done}}{Q_{absorbed}}=\frac{300,000}{1800000}=\frac{1}{6}<\frac{19}{20}=1-\frac{T_{cold}}{T_{hot}}=\eta_{Carnot}$ so it is still not a reversible cycle, right?

You didn't use the correct value for the work done by the gas for the cycle. You used the value for just the part of the cycle C → A. Otherwise, OK.

 

[lorenz0]

You didn't use the correct value for the work done by the gas for the cycle. You used the value for just the part of the cycle C → A. Otherwise, OK.

$\eta=\frac{100,000}{1800000}=\frac{1}{18}<\frac{19}{20}=1-\frac{T_{cold}}{T_{hot}}=\eta_{Carnot}.$

 

[TSny]

$\eta=\frac{100,000}{1800000}=\frac{1}{18}<\frac{19}{20}=1-\frac{T_{cold}}{T_{hot}}=\eta_{Carnot}.$

That looks good to me.