Amaelle
- 309
- 54
- Homework Statement
- Calculate the following integral (look at the image)
- Relevant Equations
- Double integrals
Greetings!
As mentionned my aim is to change the order of integral, and I totally agree with the solution I just have one question:
as you can see they have put
0<=y<=1 and 0<=x<=y^2
but would it be wrong if I put
0<=y<=1 and y^2<=x<=1?
Thank you!
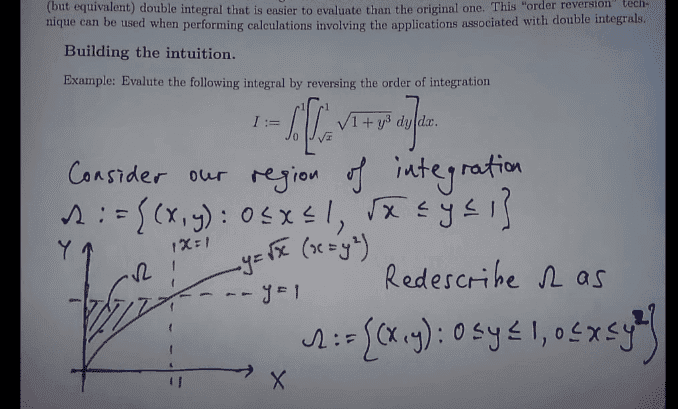
As mentionned my aim is to change the order of integral, and I totally agree with the solution I just have one question:
as you can see they have put
0<=y<=1 and 0<=x<=y^2
but would it be wrong if I put
0<=y<=1 and y^2<=x<=1?
Thank you!