- #1
AndrewEE
- 3
- 1
- Homework Statement
- Find the value of V1, V2, V3, V4
- Relevant Equations
- None
*A is the node all the way to the left above the 30V but I didn’t end up using it because I don’t think you need it since the same as 30V, right? *
I know I messed up somewhere because shouldn’t V1-4 = 30? After finding my V1 I used that value to find the other Vs and it ended up being close to -1000V
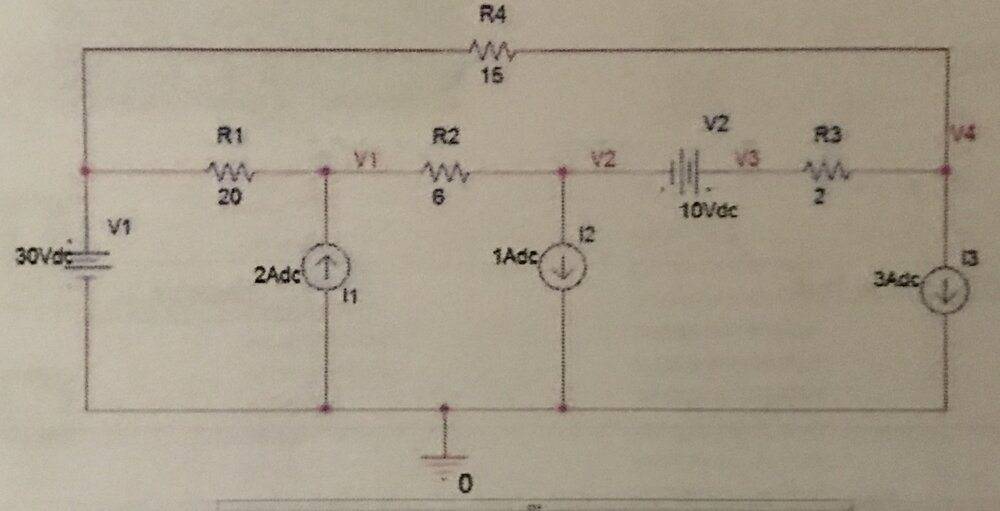

I know I messed up somewhere because shouldn’t V1-4 = 30? After finding my V1 I used that value to find the other Vs and it ended up being close to -1000V
 !
!