Collision and angular velocity

[Saitama]

Homework Statement

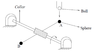
The given figure shows a mechanical system free of any dissipation. The two spheres (A and B) are each of equal mass m, and a uniform connecting rod AB of length 2r has mass 4m. The collar is massless. Right above the position of sphere A in figure is a tunnel from which balls each of mass m fall vertically at suitable intervals. The falling ballscause the rods and attached spheres to rotate. Sphere B when it reaches the position now occupied by sphere A, suffers a collision from another falling ball and so on. Just before striking, the falling ball has velocity v. All collisions are elastic and the spheres as well as the falling balls can be considered to be point masses. (Attachment 1)

(a) Find the angular velocity $\omega_{i+1}$ of the assembly in terms of {$\omega_i$, v, and r} after the ith ball has struck it.

(b) The rotating assembly eventually assumes constant angular speed $\omega^*$. Obtain $\omega^*$ in terms of v and r by solving the equation obtained in part (a). Argue how a constant $\omega^*$ does not violate energy conservation.

(c) Solve the expression obtained in part (a) to obtain $\omega_i$ in terms of {i, v, and r}.

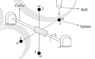
(d) If instead of a pair of spheres, we have two pairs of spheres as shown in figure below. What would be the new constant angular speed $\omega^*$ of the assembly (i.e. the answer corresponding to part (b)). (Attachment 2)

Homework Equations

The Attempt at a Solution

Let the rod be rotating with angular velocity $\omega_i$ before the next collision.

From conservation of angular momentum about centre of rod:
$$mvr+I\omega_i=I\omega_{i+1}+mv_1r$$

From conservation of energy:
$$\frac{1}{2}mv^2+\frac{1}{2}I\omega_i^2=\frac{1}{2}I\omega_{i+1}^2+ \frac{1}{2} mv_1^2$$

where I is the moment of inertia of rod+A+B and $v_1$ is the velocity of falling ball after collision.

Solving the two equations, I get:
$$\omega_{i+1}=\frac{7}{13}\omega_i+\frac{6v}{13r}$$
which is correct as per the answer key.

For part b, the question states that the angular velocity almost becomes constant.
Hence, $\omega_{i+1}=\omega_{i}$. Using this, $\omega^*=v/r$.

But what should I state for the argument? It looks odd to me that angular velocity becomes constant. The angular velocity should keep on increasing as the collisions take place.

Any help is appreciated. Thanks!

 

[voko]

What is the velocity of the sphere when the constant angular velocity is attained? What happens during its collision with the ball in that case?

 

[Saitama]

What is the velocity of the sphere when the constant angular velocity is attained?

The velocity is $v$.

What happens during its collision with the ball in that case?

I am unsure about what you ask me here. If I solve the equations, I get the resulting angular velocity to be v/r again.

Why this constant angular velocity is attained?

 

[voko]

I am unsure about what you ask me here. If I solve the equations, I get the resulting angular velocity to be v/r again.

Don't solve the equations. Just think about what happens in the reference frame of the sphere.

 

[haruspex]

If I solve the equations, I get the resulting angular velocity to be v/r again.

So what is the linear velocity of the sphere when the falling ball hits it?

 

[Saitama]

Don't solve the equations. Just think about what happens in the reference frame of the sphere.

Can you please elaborate what you actually ask me here?

So what is the linear velocity of the sphere when the falling ball hits it?

The linear velocity is $v$.

 

[voko]

Can you please elaborate what you actually ask me here?

What is the velocity of the ball at the time of the collision with the sphere, relatively to the sphere?

 

[Saitama]

What is the velocity of the ball at the time of the collision with the sphere, relatively to the sphere?

Zero.

 

[voko]

And what do you think about it? You said earlier "the angular velocity should keep on increasing as the collisions take place". Does that sound plausible if the collision happens at zero relative velocity?

 

[Saitama]

And what do you think about it? You said earlier "the angular velocity should keep on increasing as the collisions take place". Does that sound plausible if the collision happens at zero relative velocity?

But my question is how this angular velocity even attained? The collisions keep on taking place, how do we know that at some point of time the collisions take place with zero relative velocity?

Doing the maths is easy but when it comes to explaining the things, I am completely blank. :(

 

[voko]

But my question is how this angular velocity even attained? The collisions keep on taking place, how do we know that at some point of time the collisions take place with zero relative velocity?

First of all, is the situation that $\omega_i > v/r$ for any $i$ compatible with the model? Can there be a collision in such a situation?

Second, if $\omega_i < v/r$, what happens at the next collision? Does the system it gain or lose momentum?

What happens if that goes on for a very long time?

 

[Saitama]

First of all, is the situation that $\omega_i > v/r$ for any $i$ compatible with the model? Can there be a collision in such a situation?

No, the collisions will never take place.

Second, if $\omega_i < v/r$, what happens at the next collision? Does the system it gain or lose momentum?

The system gains momentum.

What happens if that goes on for a very long time?

As the collisions take place for a very long time, $\omega_i$ will tend to $v/r$.

After that there is no momentum transfer to the system, right?

 

[voko]

The system gains momentum.

Can you show that if $\omega_i < v/r$ then $\omega_{i + 1} < v/r$ and $\omega_{i + 1} > \omega_i$?

What does that imply?

 

[Saitama]

Can you show that if $\omega_i < v/r$ then $\omega_{i + 1} < v/r$ and $\omega_{i + 1} > \omega_i$?

$$\omega_i< \frac{v}{r} \Rightarrow \frac{7}{13}\omega_i<\frac{7}{13}\frac{v}{r} $$
$$\Rightarrow \frac{7}{13}\omega_i+\frac{6}{13}\frac{v}{r}<\frac{v}{r} \Rightarrow \omega_{i+1}<\frac{v}{r}$$

But how do I show $\omega_{i+1}>\omega_i$?

 

[voko]

Consider $\omega_{i + 1} - \omega_i$.

 

[Saitama]

Consider $\omega_{i + 1} - \omega_i$.

$$\omega_{i+1}-\omega_i=\frac{7}{13}\omega_i+\frac{6}{13}\frac{v}{r}-\omega_i=\frac{6}{13}\left(\frac{v}{r}-\omega_i\right)>0$$
$$\Rightarrow \omega_{i+1}>\omega_i$$

 

[voko]

Very well.

Is there anything still unclear?

 

[Saitama]

Very well.

Is there anything still unclear?

No, thank you very much voko. :)

Part 3 is easy. I have solved it and reached the correct answer.

Is the system present in part 4 similar to the system originally given? The answer states that $\omega^*$ for this system is also $v/r$. I don't think I have to make the equations again and solve (which I can easily do). The final answer is same as before so I guess the examiner wants us to provide some reasoning. How should I start with this one?

 

[voko]

The reasoning is again along the lines of #9 and #11.

Imagine you have a rotating drum in front of you. You use your hands to spin it up. Can you ever make it spin faster than your hands can move? Does that in any way depend on the moment of inertia of the drum?

 

[Saitama]

Imagine you have a rotating drum in front of you. You use your hands to spin it up. Can you ever make it spin faster than your hands can move?

No.

Does that in any way depend on the moment of inertia of the drum?

Yes, less moment of inertia implies faster spinning.

So the system in part 4 has a higher moment of inertia and it would take more time to reach $\omega^*$, right?

But I am unsure what would I write in the answer sheet. Should I simply state the system is identical to the given original system except it takes more time to reach $\omega^*$ due to higher moment of inertia?

 

[voko]

I would not say "identical", but "analogous" should be good.

Or you could try and prove that property formally for a system with an arbitrary moment of inertia.

 

[Saitama]

I would not say "identical", but "analogous" should be good.

Or you could try and prove that property formally for a system with an arbitrary moment of inertia.

Thank you very much for the help voko!

 

[sarthak7]

Just see the solution for this problem in INPhO-12 solutions...