Collision and time to stop a block

[Happiness]

[Moderator note: Misplaced homework moved from General Physics, so no template shown]

For the part on finding the total time the block spend moving, the answer is $\frac{v_0}{\mu g}$ (attached below).

I get a different answer if I use the impulse-momentum theorem:

The total change in momentum $\Delta p=-mv_0$. The force $F$ slowing the block down is always $-\mu Mg$. Thus the time $t=\frac{\Delta p}{F}=\frac{mv_0}{\mu Mg}$.

Q1: Why are the answers different?

Also, I believe the time for the case where the ball sticks to the block on the first hit should be $\frac{\Delta p}{F}=\frac{mv_0}{\mu (M+m)g}$, because the force on the ball-block combined mass is $-\mu (M+m)g$. Q2: Am I right?

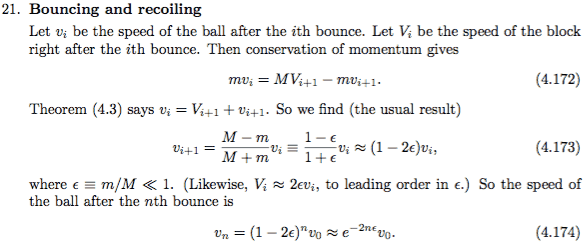
EDIT: I've found the answer to my first question. It is not correct to use the total change in momentum $\Delta p=-mv_0$ to find time $t=\frac{\Delta p}{F}$.

 

[Simon Bridge]

Well done.
You were using the wrong force in the impulse/momentum theory.

Per your second question: please show your reasoning.

 

[Happiness]

Well done.
You were using the wrong force in the impulse/momentum theory.

Per your second question: please show your reasoning.

 

[Happiness]

Per your second question: please show your reasoning.

The weight of the combined mass is $(M+m)g$. Thus the friction is $-\mu (M+m)g$.

 

[haruspex]

You were using the wrong force in the impulse/momentum theory.

I would have said the error was in overlooking the additional rightwards momentum gained from each bounce off the wall.

The weight of the combined mass is $(M+m)g$. Thus the friction is $-\mu (M+m)g$.

This is a bit awkward. It depends how you interpret the ball sticking to the block. If it sticks in such a way that the force between them is purely horizontal then you need to consider the friction on each separately, and we are told the ball has no friction.

 

[Simon Bridge]

@haruspex ; I won't always respond with a list of everything that is wrong when asked "what's wrong with this?". I'll try to point the querant in a direction that will reveal what is going on.

I'm not really clear on Happiness' reasoning throughout...

ie. the interpretation that the ball sticks to the block in such a way as to increase friction for the combination is neither here nor there for the use of the impulse-momentum formula. It's sort-of a side note. Here the impulse delivered to the m+M combination is the change in momentum ... we imagine momentum is conserved though so the momentum of the ball and of the block remains the same in total. So I wanted to see reasoning, that happiness was using, around that.

The ball momentum does change though ... since mu=(m+M)v and v<u so the impulse would be maybe give $m(u-v) = (m+M)a\Delta t$ or something?
It looks like happiness is saying that (m+M)a = \mu(m+M) or something ... ie the force on the block etc is the friction. But friction is not the only force acting on the block ... for a short time the impulse force acts on the block too since: $I=\int_0^{\Delta t}F_{I}\;dt = \Delta p_{ball}$ or whatever the actual reasoning is.
But whatever you use, that has to be the initial accelerating impulse, not the friction slowing the block ... so there is quite a lot to draw happiness' attention to ... and that worthy is right there reading this while I talk like he isn't... so: hi @Happiness , how you doing?

Can you see where there may be some concerns?
You seem to be equating the time part of the specific impulse with the time it takes the block to stop, but the impulse force is not applied during the entire motion of the block, and you seem to have equated the impulse force to the friction. You also don't account, in Q1, for the fact the wall is delivering 2p momentum to the right at each collision. In short: what is wrong with using the impulse/momentum relation here is "everything".

It looks to me like you are doing physics by trying to guess the right equations and just plugging numbers in.
You are discovering that this is not going to work. ie. the $\Delta t$ in two different equation may be referring to two different changes in time for different contexts. It is important to understand the equations you are using as well.

 

[haruspex]

I won't always respond with a list of everything that is wrong when asked "what's wrong with this?

Me neither, but Happiness had specifically asked what was wrong with the reasoning. If I reverse-engineer the reasoning from the equation, I believe it's this: time block spent moving=(momentum lost by system)/(force of friction on system while block moves). The error in that is that it ignores the impulses from the wall at each bounce.

ie. the interpretation that the ball sticks to the block in such a way as to increase friction for the combination is neither here nor there for the use of the impulse-momentum formula.

Sure, but my comment here was in respect of Happiness' second question, namely, why is the frictional force in the sticking case still μMg and not μ(M+m)g.

 

[Simon Bridge]

@haruspex fair enough and points well made. :)