- #1
ishkutay
When a photoelastic material experiences stress, its internal structure changes such that a position-dependent birefringence occurs. This means that the material effectively becomes a wave-plate, changing the polarization of incident light. It has long been known that when placed between two crossed polarizers (polarizers at 90° with respect to each other), isochromatic lines appear that indicate areas experiencing the same stress.
From my understanding, summarized above, I know these isochromatic lines have the same, rotated polarization... but why are the colors from isochrome to isochrome different?! Color, of course, is a frequency-dependent phenomenon, and I don't see how varying polarizations lead to varying colors.
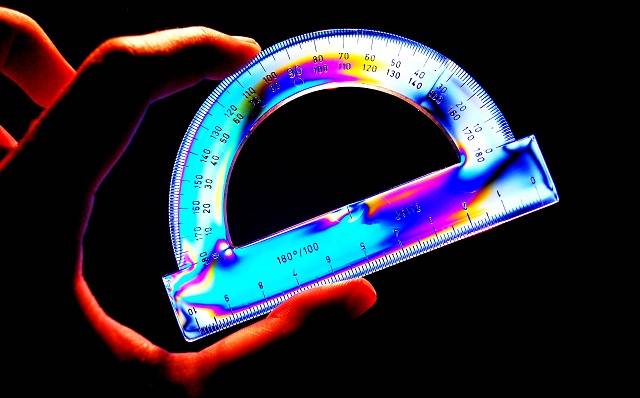
From my understanding, summarized above, I know these isochromatic lines have the same, rotated polarization... but why are the colors from isochrome to isochrome different?! Color, of course, is a frequency-dependent phenomenon, and I don't see how varying polarizations lead to varying colors.
Attachments
Last edited by a moderator:
