Combined loading, where is the neutral axis?

[morpheus343]

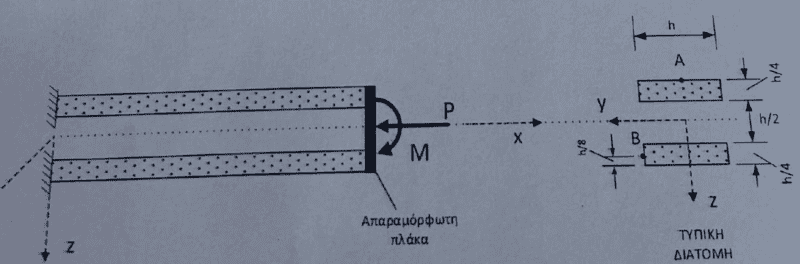
Homework Statement

distribution of stress along z, principal stress at point A.

Relevant Equations

σA=N/A+-M*y/I

Left side is fixed and right side is held together by a non deformable plate. There are two members with space inbetween. My question is when i want to calculate the stress due to bending moment at point A, which is shown in the crossection, where is the neutral axis? Is it at the middle of the whole thing, where the dotted line is (at the height of the force P), or do i take each member (top and bottom) as individuals and assume the neutral axis at the middle of each beam (h/4*1/2), so the top beam is in tension and the bottom in compression.

 

[Chestermiller]

You would do the latter.

 

[morpheus343]

If i were to calculate the maximum shear forces at point B and their orientations, given only the value of M and P, would i just use the stress transformation formulas and use as σx the axial stress since the bending stress at point B is 0 (as it is at the neutral axis) and τxy=0 ? I am guessing i cannot calculate the transverse shear using τ=VQ/It since i am not given the vertical force V
thank you for your response

 

[Chestermiller]

It seems to me there is no vertical force, only constant bending moments.

 

[erobz]

For (A) I would apply $\frac{P}{2}$ to each section for the normal compressive stress, but I would apply the bending moment to the entire composite section, and then evaluate the stress at $y = \frac{1}{2}h$. In my estimation the bending stress must be a function of the separation distance between the members. Do you have an answer key?

 

[morpheus343]

For (A) I would apply $\frac{P}{2}$ to each section for the normal compressive stress, but I would apply the bending moment to the entire composite section, and then evaluate the stress at $y = \frac{1}{2}h$. In my estimation the bending stress must be a function of the separation distance between the members. Do you have an answer key?

I do not. So it is possible to do it both ways (individual members/ the entire section) or is one of them wrong? What would the moment of inertia be for the entire section? I=(1/12*h*h^3)-(1/12*h*h/2)?

 

[erobz]

I do not. So it is possible to do it both ways (individual members/ the entire section) or is one of them wrong?

I think the entire lower beam is in compression and the entire upper beam in tension from the bending stress induced by the moment. You can imagine that this structure will become effectively stiffer as we spread the individual beams apart. I see that others have told you to use the N.A. for each beam independently. So I'm not sure if I'm correct about this. The way I see it the beams are not independent. The are effectively a single member, tied together by that "non-deformable plate" and the wall. Evaluating the stresses independently seems to not include any of the stiffening effect and reduction of stress caused by the separation distance between members. I don't mean to confuse you, so maybe someone else will chime in about the resolution of this.

What would the moment of inertia be for the entire section? I=(1/12*h*h^3)-(1/12*h*h/2)?

Not quite. You need to apply the parallel axis theorem to the individual members.

$$ I_{x'x'} = I_{xx} + A d^2 $$

 

[Lnewqban]

Welcome, @morpheus343 !

Consider the phrase “non deformable plate” to be key in this case.
As the plate can’t adopt the form of a letter S, top and bottom should be helping each other to resist external loads.
I would use only the middle vertical location of the beam formed by top and bottom members as the neutral axis.

What do you think the distribution of stress along the z axis and the principal stress at point A should be?

 

[morpheus343]

Welcome, @morpheus343 !

Consider the phrase “non deformable plate” to be key in this case.
As the plate can’t adopt the form of a letter S, top and bottom should be helping each other to resist external loads.
I would use only the middle vertical location of the beam formed by top and bottom members as the neutral axis.

What do you think the distribution of stress along the z axis and the principal stress at point A should be?

So N.A should be at h/2 and top beam is in tension while the bottom is in compression.
σA=-(P/2)/A + M*c/I which would also be the principal stress at A since σy=0 , τxy=0

 

[erobz]

So N.A should be at h/2 and top beam is in tension while the bottom is in compression.
σA=-(P/2)/A + M*c/I which would also be the principal stress at A since σy=0 , τxy=0

N.A. is at the origin of the coordinates. $c$ will be evaluated at $(A)$. $I$ is the M.o.I. of the composite beam. Think about whether the normal stresses add constructively, or destructively in a particular beam based on the sense of the applied moment ( which you seem to understand), and no other loads implies these are the principal stresses.

 

[Juanda]

Because the beam is fixed at the left side and it's held together by an undeformable plate at the right side you can solve the problem as a typical beam. Just with a little special-looking cross-section. You could imagine this beam being a $I$ profile where the web is just very thin but somehow the cross section is held together.

It's often useful to divide the acting forces and later add them back together to get the full picture. There are a few conditions that must be met for this to be possible but there's no point in discussing that now.

Acting moment M.
Since the cross-section is symmetric and the material of both beams is the same, the neutral axis will be in the center. It might look weird that it falls in an empty area but it's possible because of the plates fixed at both ends. It's similar to the center of mass of an empty circle that will be at a point where there is no mass.
The actual neutral line will be displaced due to the compression acting but we'll see that later when we add the effects of the two forces.
From your diagram, the neutral line is at $z=0$.
Here is some additional reading for the neutral axis (notice the book didn't use the same coordinates as your problem).

MECHANICS OF MATERIALS
BARRY J. GOONO / JAMES M. GERE

Secondly, the moment of inertia of the section will be $\int_{A}z^2dA$. Since they are just two blocks, you can either use the parallel axis theorem @erobz mentioned in #7 or you can subtract the moment of the empty cross-section from the full cross-section kind of how you would do it to find the area of that surface. I checked it for this area and it gives the same result ($\frac{7}{96}H^4$) using both methods. However, I'm not certain if that's just a coincidence because of all the symmetries in that cross-section or if it's a general result. Maybe @erobz knows more about that. If we can't find a confirmation, I'd proceed with the parallel axis theorem for general cases as he suggested.
Now that the neutral line's position, moment of inertia of the cross-section, and acting moment are known it is then possible to find the stress $$\sigma_x(z)=-\frac{M_yz}{I_y}$$ where $M>0$ so for $z<0$ (above the neutral line from your picture) the normal stress is positive (causing tensions).

Tension is 0 where there is no material. I used 00 to indicate that there is no tension because there is no material and that's also the neutral line that would be coming out of the screen.Acting force P
For the compression caused by $P$, it's much simpler. It'll just be the acting force divided by the total area so it'll be
$$\sigma_x=\frac{P}{A}$$ where $P$ is negative.

Finally, it's necessary to check the total effect of the combination of both.

Which gives the same "shape" but it is displaced due to the effect from $P$. As you can see, that displaces the neutral line. That will result in the lower side of the composed beam failing first because both $M$ and $P$ are causing compression on it.

Curiously enough, for materials that behave similarly in compression and tension (things like metals for example that are considered when starting these subjects) adding $P$ always has a negative impact. However, there are materials that are awful at resisting tension but are pretty good at resisting compression (concrete is the most common example). For those materials, you can see how the effect from $P$ can be positive in that scenario because the tension is reduced and the increment in compression can be resisted by the material.
Cycling loads can be another example where $P$ could be beneficial and I'm sure there are many more but those are the ones that came to my mind now.

 

[Lnewqban]

So N.A should be at h/2 and top beam is in tension while the bottom is in compression.
σA=-(P/2)/A + M*c/I which would also be the principal stress at A since σy=0 , τxy=0

The top member could be either in tension or compression, depending on the magnitudes of the external force P and the point moment M.
The assembly would be working as a column for the case of high P and low M.
It would be working as a beam for the opposite case.