Combined Rotation and Translation of a Rectangular Plate

[xkcda]

Homework Statement

A rectangular plate of mass 10 kg is at rest.It has a length of 4 meter and width of 3 meter.What is the minimum velocity required to make it rotating freely?

Relevant Equations

$I= \frac {M(l^2+b^2)} {12}$
$E=mgh$
$E=\frac {MV^2} {2}$

I tried to solve it using the work-energy theorem.The work done to make it stand on its one vertex should be equal to the change in its kinetic energy.

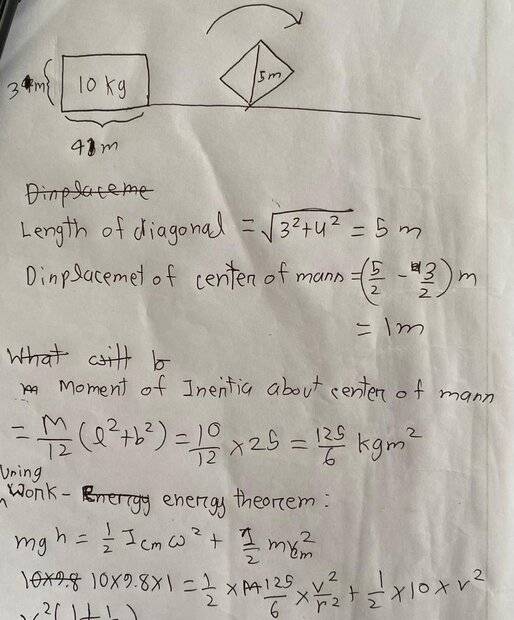
I am confused what will be the value of radius here? I have seen formula of kinetic energy for rolling of circular objects.Can anyone please derive a formula for kinetic energy of a rolling rectangular plate?

 

[haruspex]

What is the minimum velocity required to make it rotating freely?

I have no idea what that means.
Judging from your diagram and working, the idea is to give it enough angular velocity from a position where it is lying on its long edge that it will turn right over onto its short edge. Is that it?

 

[xkcda]

I have no idea what that means.
Judging from your diagram and working, the idea is to give it enough angular velocity from a position where it is lying on its long edge that it will turn right over onto its short edge. Is that it?

enough linear velocity

 

[Nugatory]

enough linear velocity

No amount of linear velocity will change the orientation of the object or make it rotate freely. Can you post the exact wording of your problem?

 

[xkcda]

No amount of linear velocity will change the orientation of the object or make it rotate freely. Can you post the exact wording of your problem?

The term I use is tangential velocity.Rotational or circular motion may require a minimal linear velocity.For instance, the bare minimum speed necessary to finish a loop.
Note: I am unable to provide the exact wording because this problem is written in my mother tongue.

 

[hutchphd]

I think the only interpretation is to suppose that the front edge hits a small protuberance elastically. Then the question makes sense and the problem can be solved simply using conservation of energy.
For the minimum speed the object will rise to a balance point with no motion so $$v^2 =2gh$$ at h=0.5m (the CM elevation). Only higher speeds require moment of inertia explicitly.

 

[Lnewqban]

Homework Statement: A rectangular plate of mass 10 kg is at rest.It has a length of 4 meter and width of 3 meter.What is the minimum velocity required to make it rotating freely?

I would do the calculation in reverse.
Balance the body over its corner, and let it fall over.
The tangential velocity with which the rotating corner is hitting the ground should be the minimum value that you are looking for.

 

[berkeman]

Note: I am unable to provide the exact wording because this problem is written in my mother tongue.

Google Translate: https://www.google.com/search?client=firefox-b-1-e&q=google+translate

 

[xkcda]

Google Translate: https://www.google.com/search?client=firefox-b-1-e&q=google+translate

I can translate better than google

 

[haruspex]

I can translate better than google

In terms of your algebra in post #1, the appropriate velocity is the initial instantaneous velocity of the mass centre of the plate. Note that this is not vertical.
Correspondingly, the rotation is about the fixed corner, so the radius is the distance to there.
But whether that is the velocity the original question asks for is unclear. A Google translation might help even if, overall, it is inferior to your own.
For one thing, it might produce correct English, which yours is not.