member 731016
- Homework Statement
- Please see below
- Relevant Equations
- Please see below
For this,
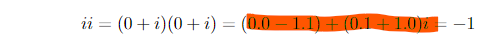
I am very confused how they got the expression in orange to become -1. Can someone please explain what happened here?
Many thanks!
I am very confused how they got the expression in orange to become -1. Can someone please explain what happened here?
Many thanks!