- #1
ktiniatros
- 2
- 0
Hi,
I got a little rusty in the basics.. Need to compute the following force f.
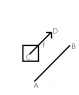
I know the coordinates of A, B and C. Segments AB and CD are parallel. So I need to find to compute the coordinates D of the force f.PS: Don't mind of the size of the square, where the force is applied. I consider it as a spot, I don't care if it has size.
I got a little rusty in the basics.. Need to compute the following force f.
I know the coordinates of A, B and C. Segments AB and CD are parallel. So I need to find to compute the coordinates D of the force f.PS: Don't mind of the size of the square, where the force is applied. I consider it as a spot, I don't care if it has size.
Attachments
Last edited: