Computing Ricci Tensor Coefficients w/ Tetrad Formalism

[snypehype46]

TL;DR Summary

Computing Ricci tensor coefficients using the tetrad formalism

I'm reading "Differentiable manifolds: A Theoretical Physics Approach" by Castillo and on page 170 of the book a calculation of the Ricci tensor coefficients for a metric is illustrated. In the book the starting point for this method is the equation given by:

$$d\theta^i = \Gamma^i_{[jk]} \theta^j \wedge \theta^k$$

where $\theta^i$ are 1-forms.

Then the book proceeds to calculate the coefficients for the following metric:

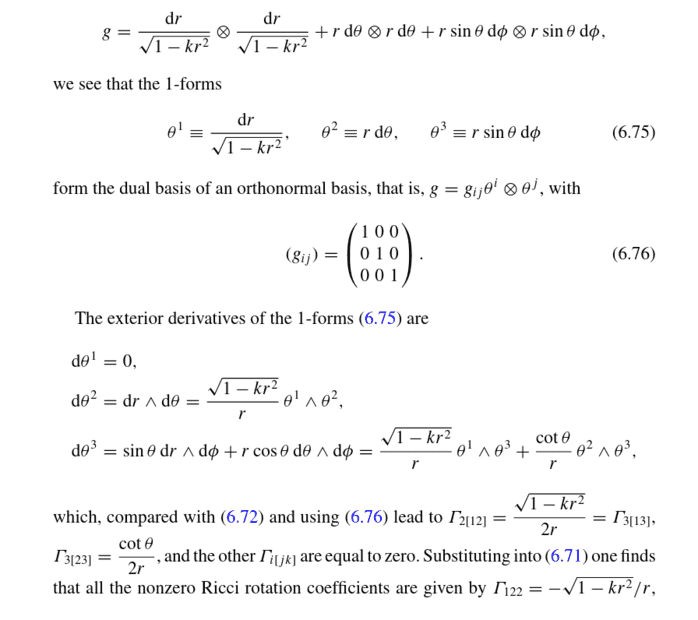
What I don't quite understand is why is the coefficient for $\Gamma_{2[12]}$ is given by $\frac{\sqrt{1-kr^2}}{2r}$. From the second equation I would have guessed the coefficient is actually $\frac{\sqrt{1-kr^2}}{r}$, so why do we need to divide by 2? From the second equation I would read $$d\theta^2 = \Gamma_{[12]}^2 \theta^1 \wedge \theta^2$$.

Also I'm not sure how the book actually computed the term for $\Gamma^3_{[13]}$.

 

[martinbn]

There is summation in the formula

$d\theta^2 = \Gamma^2_{[jk]} \theta^j \wedge \theta^k=\cdots+\Gamma^2_{[12]} \theta^1 \wedge \theta^2+\Gamma^2_{[21]} \theta^2 \wedge \theta^1+\cdots=\cdots+2\Gamma^2_{[12]} \theta^1 \wedge \theta^2+\cdots$

 

[snypehype46]

@martinbn what is the meaning of the dots?

 

[martinbn]

@martinbn what is the meaning of the dots?

Just the other terms with indeces not 1 and 2.

 

[snypehype46]

Ok I see, so for the term $\Gamma^3_{[13]}$, we used the last equation. My point of confusion is why we don't care about the factor involving $\theta^2 \wedge \theta^3$

 

[romsofia]

I'm going to be explicit!

$$d\theta^i = \Gamma^i_{jk} \theta^j \wedge \theta^K$$
$$d\theta^3 = \Gamma^3_{11} \theta^1 \wedge \theta^1 + \Gamma^3_{12} \theta^1 \wedge \theta^2 + \Gamma^3_{13} \theta^1 \wedge \theta^3$$ $$+ \Gamma^3_{21} \theta^2 \wedge \theta^1 + \Gamma^3_{22} \theta^2 \wedge \theta^2 + \Gamma^3_{23} \theta^2 \wedge \theta^3$$ $$ + \Gamma^3_{31} \theta^3 \wedge \theta^1 + \Gamma^3_{32} \theta^3 \wedge \theta^2 + \Gamma^3_{33} \theta^3 \wedge \theta^3$$

Reducing this down (which property of exterior derivatives did i use to reduce to this point?), we get:
$$d\theta^3 =\Gamma^3_{12} \theta^1 \wedge \theta^2 + \Gamma^3_{13} \theta^1 \wedge \theta^3$$ $$+ \Gamma^3_{21} \theta^2 \wedge \theta^1+ \Gamma^3_{23} \theta^2 \wedge \theta^3$$ $$ + \Gamma^3_{31} \theta^3 \wedge \theta^1 + \Gamma^3_{32} \theta^3 \wedge \theta^2$$

Now, we know that $d\theta^3 = \frac{\sqrt{1-kr^2}}{r} \theta^1 \wedge \theta^3 + \frac{\cot \theta}{r} \theta^2 \wedge \theta^3$

Match the same with the same. Which terms involve $\theta^1 \wedge \theta^3$? You're not ignoring the $\theta^2 \wedge \theta^3$ terms, it's just that $\Gamma^3_{13}$ doesn't have any $\theta^2 \wedge \theta^3$ terms!

Also, some food for thought, what can you conclude about $\Gamma^3_{12}, \Gamma^3_{21}$ from this equation?

 

[haushofer]

A quickie: factors of 2 and antisymmetrization [...] , what's the book's convention? Weight one (anti)symmetrization?

 

[stevendaryl]

I have never worked in a non-coordinate basis before, so I'm having trouble with the basic equation:

$d \Theta^i = \Gamma^i_{|jk|} \Theta^j \wedge \Theta^k$

Is $\Gamma^i_{jk}$ defined for a non-coordinate basis via:

$\nabla_{e_i} e_j = \Gamma^k_{ij} e_k$

If so, then it seems to me that if $e_\mu$ is a coordinate basis, with corresponding connection $\Gamma^\lambda_{\mu \nu}$, and $e_i$ is some other basis (coordinate or not), then the relationship between the $\Gamma$s is given by:

$\Gamma^k_{ij} = \Lambda^\nu_i \Lambda^k_\mu \partial_\nu \Lambda^\mu_j + \Lambda^k_\mu \Lambda^\nu_i \Lambda^\lambda_j \Gamma^\mu_{\nu \lambda}$

where $\Lambda^\mu_i$ is the coordinate transformation matrix, and $\Lambda^i_\mu$ is its inverse.

On the other hand, in the covector basis,
$e^k = \Lambda^k_\mu e^\mu = \Lambda^k_\mu d x^\mu$

Taking exterior derivatives of both sides gives:

$d e^k = d(\Lambda^k_\mu d x^\mu) = (\partial_\nu \Lambda^k_\mu) d x^\nu \wedge dx^\mu$ (because $d d x^\mu = 0$)

Re-expressing in terms of the noncovariant basis $e^i$ gives:

$d e^k = \Lambda^\nu_i \Lambda^\mu_j (\partial_\nu \Lambda^k_\mu) e^i \wedge e^j$

So that's not the same thing as $d e^k = \Gamma^k_{|ij|} e^i \wedge e^j$. Is it?

 

[vanhees71]

In holonomic bases ("coordinate bases") in a space without torsion (as in standard GR) the connection coefficients are the Christoffel symbols, which are symmetric in the lower components.

That's different for (pseudo)-orthonormal bases of course.