vcsharp2003
- 913
- 179
- TL;DR Summary
- Is degree of freedom just an independent term/variable/coordinate or the number of independent terms/variables/coordinates?
I am seeing conflicting definitions of degree of freedom in my textbook. If I look at the definition given as per screenshot below then it is the number of independent terms/variables/coordinates used to define the energy of a molecule. But, if I look at the statement of Equipartition of energy that is given below the definition, then it seems that degree of freedom is any one of independent terms/variables/coordinates used to get energy of a molecule.
I think degree of freedom should just be the independent term/variable/coordinate.
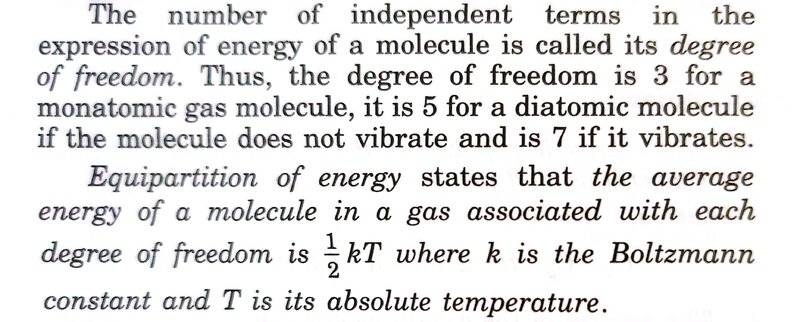
I think degree of freedom should just be the independent term/variable/coordinate.