Confused About Boundary Conditions for ##y## and ##x##

[chwala]

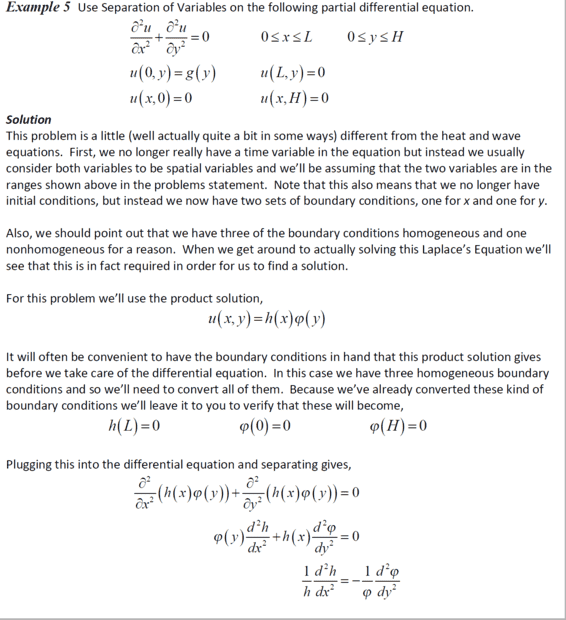
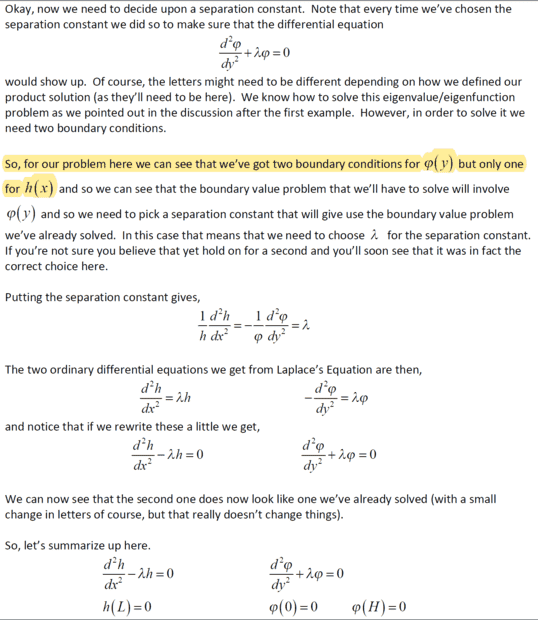
Homework Statement

Kindly see attached.

Relevant Equations

separation of variables and basic knowledge on boundary and initial conditions

I am going through this notes, i can follow quite well...my only issue is on the highlighted part...i thought that we had two boundary conditions for $y$ ( of which one of them is non homogenous) and two boundary conditions for $x$( of which both are homogenous)...kindly clarify on this part...

 

[chwala]

Here is my working on the given homogenous boundary conditions,
Let $U(x,y) = X⋅Y$
1. Given, $u(x,0)= 0$, then it follows that,
$0=X(x) Y(0)$ →$Y(0)=0$

2. Given, $u(L,y)= 0$, then it follows that,
$0=X(L) Y(y)$ →$X(L)=0$

3. Given, $u(x,H)= 0$, then it follows that,
$0=X(x) Y(H)$ →$Y(H)=0$

The remaining steps to solution will follow accordingly...

 

[Orodruin]

You have two homogeneous boundary conditions on y and one on x. The second boundary condition on x is inhomogeneous.

 

[chwala]

You have two homogeneous boundary conditions on y and one on x. The second boundary condition on x is inhomogeneous.

Thanks i got a little mixed up there,...from my opening remarks on post $1$, the boundary conditions for $x$ and $y$ ought to be the way you've indicated...and not other way round. Cheers...

 

[chwala]

...also for the problem below, which is quite related to the original post;

Note
Kindly note that i share this so that when it comes to solving pde related problems in future then atleast you guys will have an understanding of my approach )...cheers guys.

Ok here, we have,
$u_t=k u_{xx} - u$ given the initial condition, $u(x,0)= f(x)$ and boundary conditions, $u(0,t)=0$ and $-u_x(L,t)=u(L,t)$ then,

Let $u(x,t)= XT$
$u_t= XT^{'}$
$u_{xx}= X^{''}T$
on substitution to the pde, we shall have,
$XT^{'}$=$kX^{''}T-XT$
...
$\frac {T^{'}}{T}$=$\frac {kX^{''}-X}{X}$=$-λ$
$\frac {T^{'}}{T}$+$1$=$\frac {kX^{''}}{X}$=$-λ$
$→\frac {1}{k}$[$\frac {T^{'}}{T}$+$1$]=$\frac {X^{''}}{X}$=$-λ$ (two ordinary differential equations realized)...
Now when it comes to the boundary conditions, we know that,
$u(x,t)= XT$
1. $u(0,t)= 0$
→$0=X(0)T(t)$, $T(t) ≠0$ →$X(0)=0$

2. $u(L,t) + u_x(L,t)=0$
$X(L)T(t) +X^{'}(L)T(t)=0$
since$X(0)$=$0$ →$X(L)=X^{'}(L)$=$0$

bingo