member 731016
- Homework Statement
- Please see below
- Relevant Equations
- Please see below
For this problem,
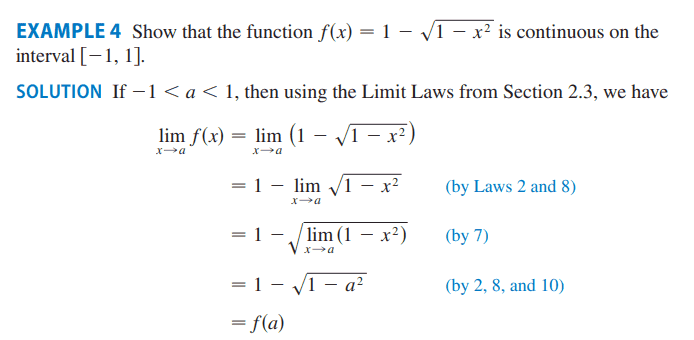
I don't understand why they are saying ##-1 < a < 1## since they are trying to find where ##f(x)## is continuous including the endpoints ##f(-1)## and ##f(1)##
Why is it not: ##-1 ≤ a ≤1##
Many thanks!
I don't understand why they are saying ##-1 < a < 1## since they are trying to find where ##f(x)## is continuous including the endpoints ##f(-1)## and ##f(1)##
Why is it not: ##-1 ≤ a ≤1##
Many thanks!
