tracker890 Source h
- 90
- 11
- Homework Statement
- I feel that the mass within the system changes over time, but this perception contradicts the solution.
- Relevant Equations
- continuity equation
Q: Why does assuming "Properties in the tank are uniform, but time-dependent" lead to the validity of
(DmDt)sys=0? Doesn't the mass within the system change over time?
reference.
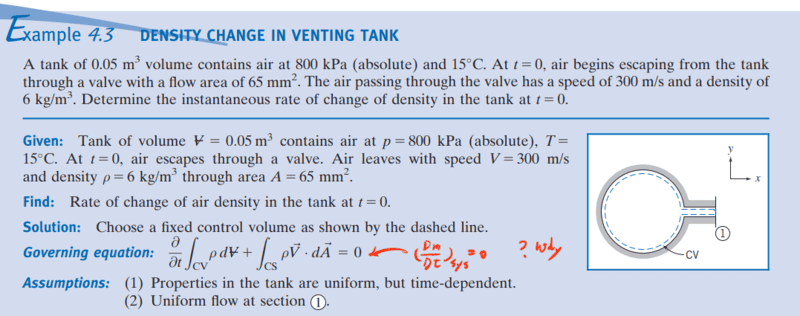
(DmDt)sys=0? Doesn't the mass within the system change over time?
reference.