vcsharp2003
- 913
- 179
- Homework Statement
- Two capacitors of ##3 \text{ } \mu \text{F}## and ##4 \text{ } \mu \text{F}## are connected so negative plate of one is attached to positive plate of the other as shown in diagram. What is the final charge on each capacitor? These capacitors were initially charged using a 6 V battery.
- Relevant Equations
- C = V /q
Also, charges should be conserved since new charges cannot be created or existing ones destroyed
I looked at the solution of this problem since its a solved problem. I am having doubts with the charges relationship as is mentioned in screenshot below. The charges ##{q_3}^{'}## and ##{q_4}^{'}## are the charges after a a state of balance is reached.
Why would the charges have the relationship as mentioned in screenshot below? I have determined ## q_3 = 18 \text{ } \mu \text{F}## and ## q_4 = 24 \text{ } \mu \text{F}## using the equation q = CV.
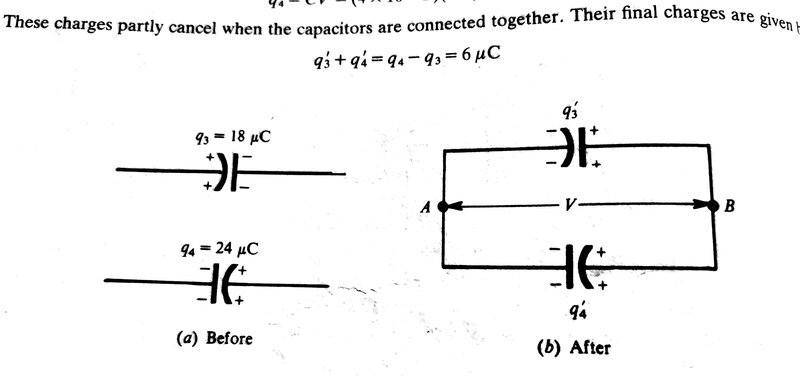
Why would the charges have the relationship as mentioned in screenshot below? I have determined ## q_3 = 18 \text{ } \mu \text{F}## and ## q_4 = 24 \text{ } \mu \text{F}## using the equation q = CV.
Last edited: