- #1
Jarek 31
- 158
- 31
- TL;DR Summary
- Both renormalization and regularization are used to remove infinities in similar situations (e.g. charged particle), but from different perspectives - is there a connection/correspondence between them?
While in QFT we remove infinite energy problem with renormalization procedure, asking e.g. "what is mean energy density in given distance from charged particle", electric filed alone would say $$\rho \propto |E|^2 \propto 1/r^4 $$
But such energy density would integrate to infinity due to singularity in zero.
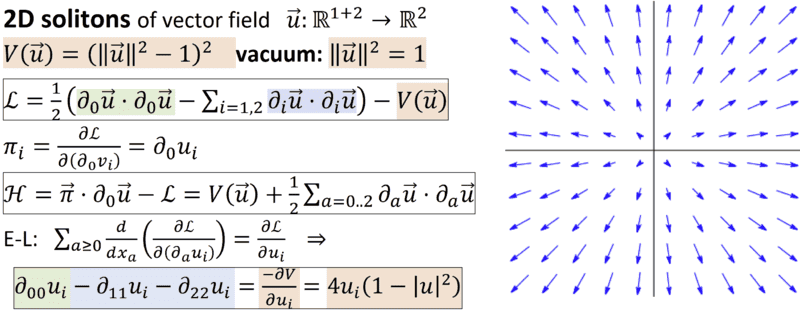
In field theories (usually classical, but they can be quantized e.g. https://arxiv.org/pdf/hep-th/0505276.pdf ) we use regularization to remove this kind of infinities, for example vector field with Higgs potential V(u)=(|u|^2-1)^2 - preferring unitary vectors, but allowing for their deformation to prevent infinity like below.
So is there a connection/correspondence between these two approaches to remove infinity of singularity - applied to the same situation like charged particle, but from different perspectives?
But such energy density would integrate to infinity due to singularity in zero.
In field theories (usually classical, but they can be quantized e.g. https://arxiv.org/pdf/hep-th/0505276.pdf ) we use regularization to remove this kind of infinities, for example vector field with Higgs potential V(u)=(|u|^2-1)^2 - preferring unitary vectors, but allowing for their deformation to prevent infinity like below.
So is there a connection/correspondence between these two approaches to remove infinity of singularity - applied to the same situation like charged particle, but from different perspectives?