- #1
wudingbin
- 24
- 0
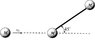
a rigid massless rod of length L joins two particles each of mass M. The rod lies on
a frictionless table, and is struck by a particle of mass M and velocity v0. After the collision, the projectile moves straight back. Find the
angular velocity of the rod about its center of mass after the collision, assuming that
mechanical energy is conserved.
i tried to solve this question using the conservation of linear momentum first.
using ((m-2m)/3m)v0 to find the speed of the mass M after collision.
(2m/3m)v0, to find the speed of the rod after collision.
translational speed of the rod = (2/3v0 - wr)
angular speed of the rod = w
hence i used the conservation of energy method to solve for w.
however, my answer is incorrect. may i know which part, i am wrong
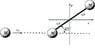
a frictionless table, and is struck by a particle of mass M and velocity v0. After the collision, the projectile moves straight back. Find the
angular velocity of the rod about its center of mass after the collision, assuming that
mechanical energy is conserved.
i tried to solve this question using the conservation of linear momentum first.
using ((m-2m)/3m)v0 to find the speed of the mass M after collision.
(2m/3m)v0, to find the speed of the rod after collision.
translational speed of the rod = (2/3v0 - wr)
angular speed of the rod = w
hence i used the conservation of energy method to solve for w.
however, my answer is incorrect. may i know which part, i am wrong