- #1
jisbon
- 476
- 30
- Homework Statement
- A disc is spinning at 6.8 rad/s about the centre of the disc. A solid iron sphere is then attached to the edge of the disc and rotate together, find the angular velocity of the whole system now.
- Relevant Equations
- -
Not sure what to do here, except using the conversation of angular momentum. Even then, is angular momentum conserved in this case even after attaching an external object here? Else, what laws can I use to solve this problem?
Using conversation of angular momentum:
$$\dfrac {1}{2}M_{disc}R^{2}_{disc}\left( 6.8\right) =\left( M_{disc}R^{2}_{disc}W\right) +\left( \dfrac {2}{5}M_{sphere}\left( R_{disc}+R_{sphere}\right) ^{2}W\right) $$
Is this correct?
Diagram:
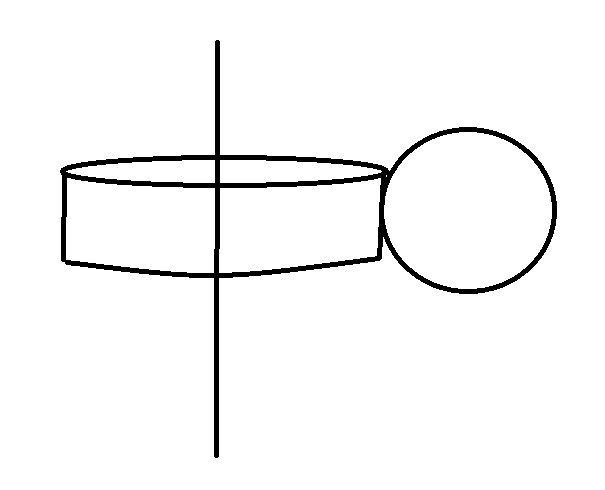
Using conversation of angular momentum:
$$\dfrac {1}{2}M_{disc}R^{2}_{disc}\left( 6.8\right) =\left( M_{disc}R^{2}_{disc}W\right) +\left( \dfrac {2}{5}M_{sphere}\left( R_{disc}+R_{sphere}\right) ^{2}W\right) $$
Is this correct?
Diagram:
