Conservation of momentum problem

[assaftolko]

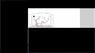
A body with a mass of M is on a friction-free horizontal floor. The shape of the body is a half circle with a radius of R. A mass of m is put at the left edge of M and is released from rest. the mass m rolls on the half circle with no friction.

Is there conservation of momentum during the movement in respect to the system?

Well, in the solution they say that for the horizontal x-axis there is, since no external forces are applied on the system in the x axis. But they say that for the vertical y-axis there is no conservation of momentum but I don't understand their explanation: They draw the forces on M and m (I uploaded this draw) for a moment where m is at something like half the distance between its start point and the bottom of the circle point. They say that because N is an internal force in the system, it doesn't affect the external impulse. And then they say that Nfloor cancells Mg and because there is no external force to cancel mg, you get that for the y-axis the external impulse isn't 0 and so there is no conservation of momentum for the y axis. I don't understand this analysis: How can they on one hand - decide to look at the system where N is an internal force, but on the other hand to determine that Nfloor cancells out Mg?? I mean if anything, Nfloor cancells out (M+m)g if we want to look at the system... Am I wrong?

 

[ehild]

The rate of change of momentum of a system is equal to the sum of the external forces.

The system consist of the big body and the small ball. The external forces are gravity and the normal force from the table. Gravity acts both on the big mass and on the ball, but the ball moves, so the centre of mas of the whole system will move in vertical direction, while the horizontal position stays stationary.

If you consider the forces separately for both bodies, the big body has Mg downward force and N_f normal force from the floor upward and N_b normal force from the ball. Nb has both vertical and horizontal components. As the big body does not change its vertical position, N_f-Mg-N_by=0 The normal force from the floor is not necessarily equal to Mg, but the net vertical force is not zero in general. (It is when the ball is ta the bottom of the circle).

ehild

 

[assaftolko]

The rate of change of momentum of a system is equal to the sum of the external forces.

The system consist of the big body and the small ball. The external forces are gravity and the normal force from the table. Gravity acts both on the big mass and on the ball, but the ball moves, so the centre of mas of the whole system will move in vertical direction, while the horizontal position stays stationary.

If you consider the forces separately for both bodies, the big body has Mg downward force and N_f normal force from the floor upward and N_b normal force from the ball. Nb has both vertical and horizontal components. As the big body does not change its vertical position, N_f-Mg-N_by=0 The normal force from the floor is not necessarily equal to Mg, but the net vertical force is not zero in general. (It is when the ball is ta the bottom of the circle).

ehild

I still don't understand how do you know that the net vertical force isn't zero (and why it is if m is at the bottom of the circle?). I mean no matter where m is - we know that for M the acceleration in the y-axis is 0 always. And so - Nfloor adjusts its size along the movement of m in order to counter the affect from Mg and the vertical component of Nb - which changes along the movement of m according to the angle Nb has with the y axis... I'm sorry but I don't understand why the net vertical force isn't 0 for the system...

 

[assaftolko]

I still don't understand how do you know that the net vertical force isn't zero (and why it is if m is at the bottom of the circle?). I mean no matter where m is - we know that for M the acceleration in the y-axis is 0 always. And so - Nfloor adjusts its size along the movement of m in order to counter the affect from Mg and the vertical component of Nb - which changes along the movement of m according to the angle Nb has with the y axis... I'm sorry but I don't understand why the net vertical force isn't 0 for the system...

never mind i think i got you thanks a lot man!

 

[ehild]

The vertical force is zero at the bottom of the circle if the ball is in rest. Otherwise it is not zero, when the ball has vertical acceleration.
Have you solved the whole problem?

ehild

 

[voko]

Imagine a ball in free fall. Is the momentum conserved?

 

[assaftolko]

Imagine a ball in free fall. Is the momentum conserved?

No, because only gravity applies (assuming there's no drag) on the ball... but here you have to consider the normal force from the floor

 

[assaftolko]

The vertical force is zero at the bottom of the circle if the ball is in rest. Otherwise it is not zero, when the ball has vertical acceleration.
Have you solved the whole problem?

ehild

Not yet I didn't have time today. Ehild I'm sorry but after thinking again I still don't understand how do you know that there's no conservation of momentum in the y-axis can you be more specific?

Why do you say on the one hand that for M: Nf-Mg-Nby=0 but on the other hand you say: ..."but the net vertical force is not zero in general".

 

[ehild]

Sorry, I somehow erased the equation for the small ball: N_by-mg=ma_y, which is not zero in general. So the total force on the whole system is N_f-(m+M)g=ma_y,
which is not zero in general. It would be zero if the ball stayed in rest at the bottom of the circle. As it can not be said that the sum of the external forces is zero, the momentum of the system is not conserved.


ehild

 

[assaftolko]

Sorry, I somehow erased the equation for the small ball: N_by-mg=ma_y, which is not zero in general. So the total force on the whole system is N_f-(m+M)g=ma_y,
which is not zero in general. It would be zero if the ball stayed in rest at the bottom of the circle. As it can not be said that the sum of the external forces is zero, the momentum of the system is not conserved.


ehild

Well that's a bit more understood to me, thanks. But can you even reffer to the concept "The total force on the whole system" when it's clear that there's reletive movement between the ball and M all along the way? I mean you showed that Nfloor-(M+m)g doesn't equal 0, but can I even say that Nfloor-(M+m)g is the total force on the system considering m and M don't have the same acceleration in every moment?

Also please tell me if you think the next explanation is valid: At first both M and m are at rest, so the momentum of the system in both the x-axis and in the y-axis is 0. If there was conservation of momentum in the y-axis we'd expect that for every moment along the movement, the momentum in the y-axis will be 0 for the whole system. Now it's clear that M moves only in the x direction so its momentum in the y-axis in every moment is 0. But it's also clear that m has vertical speed along the way because it changes its vertical position as it rolls on M. So if m has vertical speed and M has no vertical speed along the movement - then the net momentum for the system in the y-axis is clearly not 0 like at the beginning, hence there can be no conservation of momentum in the y axis!

 

[ehild]

Well that's a bit more understood to me, thanks. But can you even reffer to the concept "The total force on the whole system" when it's clear that there's reletive movement between the ball and M all along the way? I mean you showed that Nfloor-(M+m)g doesn't equal 0, but can I even say that Nfloor-(M+m)g is the total force on the system considering m and M don't have the same acceleration in every moment?

You can speak of the total force acting to a system of bodies and it is equal to the total mass multiplied by the acceleration of the CM (centre of mass)
The acceleration of the CM in vertical direction is (a(body)M+a(ball)m)/(m+M), but a(body)=0, so it is a(ball)m/(m+M).
That means ƩF = Nf-(m+M)g=a(ball)m.

Also please tell me if you think the next explanation is valid: At first both M and m are at rest, so the momentum of the system in both the x-axis and in the y-axis is 0. If there was conservation of momentum in the y-axis we'd expect that for every moment along the movement, the momentum in the y-axis will be 0 for the whole system. Now it's clear that M moves only in the x direction so its momentum in the y-axis in every moment is 0. But it's also clear that m has vertical speed along the way because it changes its vertical position as it rolls on M. So if m has vertical speed and M has no vertical speed along the movement - then the net momentum for the system in the y-axis is clearly not 0 like at the beginning, hence there can be no conservation of momentum in the y axis!

It is an excellent explanation!

ehild

 

[assaftolko]

You can speak of the total force acting to a system of bodies and it is equal to the total mass multiplied by the acceleration of the CM (centre of mass)
The acceleration of the CM in vertical direction is (a(body)M+a(ball)m)/(m+M), but a(body)=0, so it is a(ball)m/(m+M).
That means ƩF = Nf-(m+M)g=a(ball)m.



It is an excellent explanation!

ehild

Thanks man! I do have to say that the second explanation (which accedently crossed my mind as I woke up today) is the most simple one to understand (for me anyway), but now I also understand the logic behind your explanation, thanks!

 

[assaftolko]

The vertical force is zero at the bottom of the circle if the ball is in rest. Otherwise it is not zero, when the ball has vertical acceleration.
Have you solved the whole problem?

ehild

The other questions in this problem are as follow:

1. Write the consrvation of momentum and energy equations if they exist.
2. What is the velocity of each mass whem m is at the lowest point of the circle?
3. Will m get to the top right edge of M? If so what would be the velocity of m? If not, What would be the max height m would get to in respect to the lowest point of the circle?

1. Well we've agreed (finally) that there is conservation of momentum in x and no conservation of momentum in y. The general conservation of momentum equation for the x-axis is:

mvx-MVx=0 -> mvx=MVx -> Vx=(m/M)vx

There is conservation of energy for the system because the net work done by N is 0, and the work done by Nfloor is 0 because Nfloor is perpendicular to M's movement direction. And so the conservation of energy equation is:

mgR=0.5mv^2+0.5MVx^2+mghi
where hi is the height of a general point i in respect to the bottom of the circle.

2. Using these 2 equations we'll look at the start point (A) and the bottom of the circle point (B):

Px(A)=0 Px(B)=mvx-MVx -> Vx=(m/M)vx <But at the bottom point vx=v> -> Vx=(m/M)v

E(A)=mgR E(B)=0.5mv^2+0.5MVx^2+mg*0 and so if we'll use the first equation:

mgR=0.5mv^2+0.5M*(m/M)^2*v^2 --> v=sqrt[(2gR)/(1+m/M)]

And so: Vx=(m/M)*sqrt[(2gR)/(1+m/M)]

3. Let's assume m stops at a lower point than the top right edge of M. Because at first the system had the energy of mgR, and at this lower point h<R, it's clear that if we want to maintain the conservation of energy there should be contribution of kinetic enegry to this lower potential energy point, at which m stops. But - if m stops than for this moment in time it has the same velocity as M does (when m stops at its max height point, it's at rest relative to M but not to the ground). But from the conservation of momentum we get that for every moment Vx=(m/M)vx, and if Vx=vx in this max height point, then it's clear that Vx=vx=0 (Assuming M doesn't equal to m). But if Vx=vx=0 there can be no contribution of kinetic energy at this point. Hence - m can only stop at the top right edge of M. At this point it has mgR potential energy and it's velocity has to be zero!

Is my explanation to 3 is correct?

 

[ehild]

It is a nice explanation and I think it is correct.ehild

 

[assaftolko]

It is a nice explanation and I think it is correct.


ehild

Thanks a lot!