Continuous functions on metric spaces

[Lambda96]

Homework Statement

Show that the mapping $\phi$ is continuous

Relevant Equations

none

Hi,

I don't know if I have solved task correctly

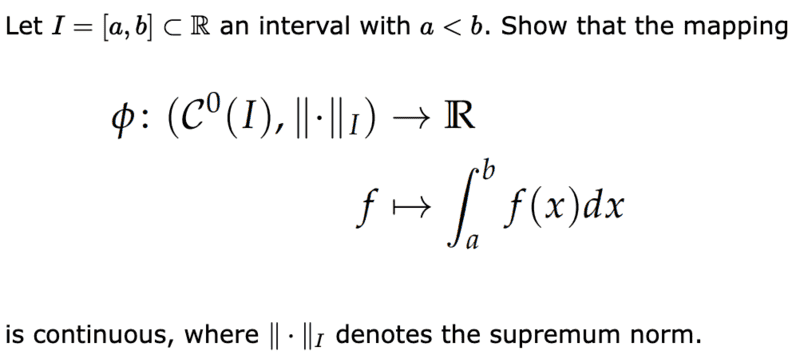
I used the epsilon-delta definition for the proof, so it must hold for $f,g \in (C^0(I), \| \cdot \|_I)$ $\sup_{x \in [a,b]} |F(x)-G(x)|< \delta \longrightarrow \quad |\int_{a}^{b} f(x)dx - \int_{a}^{b} g(x)dx |< \epsilon$

I then proceeded as follows

$|\int_{a}^{b} f(x)dx - \int_{a}^{b} g(x)dx | =|F(b)-F(a)-G(b)+G(a)|=|F(b)-G(b)+G(a)-F(a)|$

Then I used the supremum norm to estimate the above expression $|F(b)-F(a)-G(b)+G(a)|=|F(b)-G(b)+G(a)-F(a)| \le \sup_{x \in [a,b]} |F(x)-G(x)|+\sup_{x \in [a,b]} |F(x)-G(x)|+\sup_{x \in [a,b]} |G(x)-F(x)|=2\sup_{x \in [a,b]} |F(x)-G(x)|<2\delta$ it then follows that $\delta$ must be defined as follows $\delta= \frac{\epsilon}{2}$

 

[Orodruin]

You want to find a $\delta$ such that if $||f-g|| = \sup_{x \in I}|f(x)-g(x)| < \delta$ then $|\phi(f) - \phi(g)|< \varepsilon$. It seems to me that you have instead used the primitive function of $f$ and $g$ in the supremum norm.

 

[pasmith]

Hint: By basic properties of the Riemann integral, <br /> \left| \int_a^b f(x)\,dx \right| \leq \int_a^b |f(x)|\,dx \leq (b- a) \sup |f|.

 

[WWGD]

You want to find a $\delta$ such that if $||f-g|| = \sup_{x \in I}|f(x)-g(x)| < \delta$ then $|\phi(f) - \phi(g)|< \varepsilon$. It seems to me that you have instead used the primitive function of $f$ and $g$ in the supremum norm.

How do you know the space $C^0$ has a vector space structure that allows you to subtract functions? Edit: Never mind, it's a normed space, hence a vector space.
Seems you could too, just use the open set definition and verify that the inverse image of an interval is open in $C^0$ with the given norm.

 

[Lambda96]

Thank you Orodruin, pasmith and WWGD for your help

I have now used the tip from pasmith and received the following

The following must apply:
$\sup_{x \in [a,b]} |f(x)-g(x)|< \delta \longrightarrow \quad |\int_{a}^{b} f(x)dx - \int_{a}^{b} g(x)dx |< \epsilon$

Then I determined $\delta$ as follows
$$|\int_{a}^{b} f(x)dx - \int_{a}^{b} g(x)dx \le |\int_{a}^{b} |f(x)|dx - \int_{a}^{b} |g(x)|dx \le (b-a) \sup f|x|-(b-a) \sup |g(x)| = (b-a) \bigl( \sup|f(x)| - \sup|g(x)| \bigr)$$

Then $\delta$ must be $\delta = \frac{\epsilon}{b-a}$

 

[Orodruin]

Then I determined $\delta$ as follows
$$|\int_{a}^{b} f(x)dx - \int_{a}^{b} g(x)dx \le |\int_{a}^{b} |f(x)|dx - \int_{a}^{b} |g(x)|dx \le (b-a) \sup f|x|-(b-a) \sup |g(x)| = (b-a) \bigl( \sup|f(x)| - \sup|g(x)| \bigr)$$

The first inequality is incorrect.

 

[PeroK]

$$|\int_{a}^{b} f(x)dx - \int_{a}^{b} g(x)dx \le |\int_{a}^{b} |f(x)|dx - \int_{a}^{b} |g(x)|dx \le (b-a) \sup f|x|-(b-a) \sup |g(x)| = (b-a) \bigl( \sup|f(x)| - \sup|g(x)| \bigr)$$

That all looks ill-conceived to me. For example, I don't think you've corrently identified the metric that is derived from the given supremum norm.

PS as pointed out in post #2.

 

[pasmith]

|\phi(f) - \phi(g)| = \left| \int_a^b f(x)\,dx - \int_a^b g(x)\,dx \right| = \left| \int_a^b f(x) - g(x)\,dx\right| etc.

 

[Lambda96]

Thank you Orodruin, PeroK and pasmith for your help

$$ \bigg \vert \int_{a}^{b} f(x) dx - \int_{a}^{b} g(x)dx \bigg \vert= \bigg \vert\int_{a}^{b} f(x)-g(x) dx \bigg \vert \le \int_{a}^{b} |f(x)-g(x)| dx \le (b-a) \sup|f(x)-g(x)|$$


From this follows $\delta=\frac{\epsilon}{b-a}$

 

[PeroK]

Thank you Orodruin, PeroK and pasmith for your help

$$ \bigg \vert \int_{a}^{b} f(x) dx - \int_{a}^{b} g(x)dx \bigg \vert= \bigg \vert\int_{a}^{b} f(x)-g(x) dx \bigg \vert \le \int_{a}^{b} |f(x)-g(x)| dx \le (b-a) \sup|f(x)-g(x)|$$


From this follows $\delta=\frac{\epsilon}{b-a}$

That's fine as far as it goes, but you really need a sound, logical proof. All $\epsilon-\delta$ proofs, in principle, should start with "Let $\epsilon > 0 \dots$".

 

[Orodruin]

How do you know the space $C^0$ has a vector space structure that allows you to subtract functions? Edit: Never mind, it's a normed space, hence a vector space.

Even without that, it should be pretty clear that a pointwise sum of continuous functions is a continuous function. All of the vector space axioms are clearly satisfied.

Seems you could too, just use the open set definition and verify that the inverse image of an interval is open in $C^0$ with the given norm.

This is always my preferred way forward. However, the OP is most likely not aware of this definition of continuity.

 

[PeroK]

Seems you could too, just use the open set definition and verify that the inverse image of an interval is open in $C^0$ with the given norm.

This is always my preferred way forward. However, the OP is most likely not aware of this definition of continuity.

I'd like to see a simple proof in this case using that method. Or any proof, simple or otherwise, that isn't essentially just the $\epsilon-\delta$ we have already.

 

[Lambda96]

Many thanks to all of you for your help


For the above problem, I had decided to use the proof for the epsilon delta definition, as my lecturer told me that I would have to know it for the exam.

According to my professor's lecture notes, if the mapping is linear, you can also use boundedness to prove continuity

I have another problem where I have to show continuity, can I post it here or should I open a new thread?

 

[pasmith]

You should start a new thread. Note that this new question is a generalization of the question you posted in this thread.

 

[PeroK]

According to my professor's lecture notes, if the mapping is linear, you can also use boundedness to prove continuity

View attachment 345476

Do you see the close relationship between the proof of that theorem and the proof in this particular case?