member 731016
- Homework Statement
- Please see below. I have a doubt about the solution of the problem.
- Relevant Equations
- Arc length = ##R\theta##
The problem and solution are,
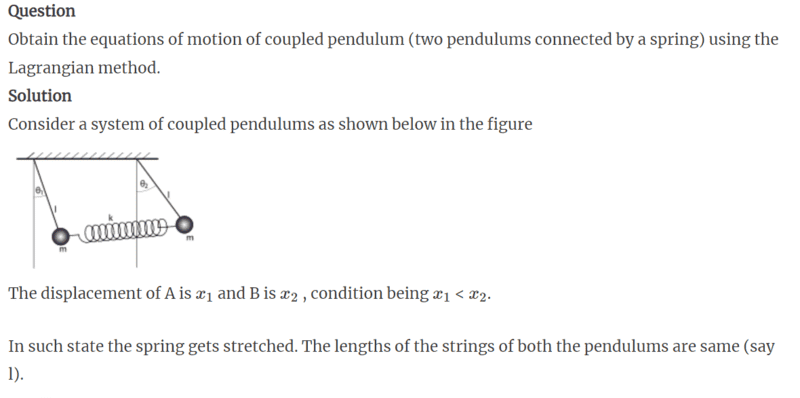


However, I am confused how the separation vector between the two masses is
##\vec x = x \hat{k} = x_2 \hat{x_2} - x_1 \hat{x_1}= l\theta_2 \hat{x_2} - l\theta_1 \hat{x_1 } = l(\theta_2 - \theta_1) \hat{k}##. where I define the unit vector from mass 2 to mass 1 as ##\hat{k}## along the spring. Does someone please know of a algebraic or geometric proof for this?
Thanks alot!
However, I am confused how the separation vector between the two masses is
##\vec x = x \hat{k} = x_2 \hat{x_2} - x_1 \hat{x_1}= l\theta_2 \hat{x_2} - l\theta_1 \hat{x_1 } = l(\theta_2 - \theta_1) \hat{k}##. where I define the unit vector from mass 2 to mass 1 as ##\hat{k}## along the spring. Does someone please know of a algebraic or geometric proof for this?
Thanks alot!