- #1
Beer-monster
- 296
- 0
Homework Statement
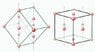
I'm calculating the distances between to nearest atoms from various planes an a cubic crystal. For example I know that the distance from the [111] plane to the nearest atom is for a fcc lattice
[tex] d=\frac{\sqrt{3}a}{3} [/tex]
What I'm unsure about is where the 1/3 factor comes from. I know it has to do with the position of the plane to the atom but the actually calculation escapes me.
Can anyone explain it?
Also when I draw the [110] plane for fcc lattice and try to work out the nearest atom in that direction, I can't find the nearest atom. There doesn't seem to be anything in that direction. Where am I going wrong?
Thanks