- #1
annamal
- 387
- 33
- Homework Statement
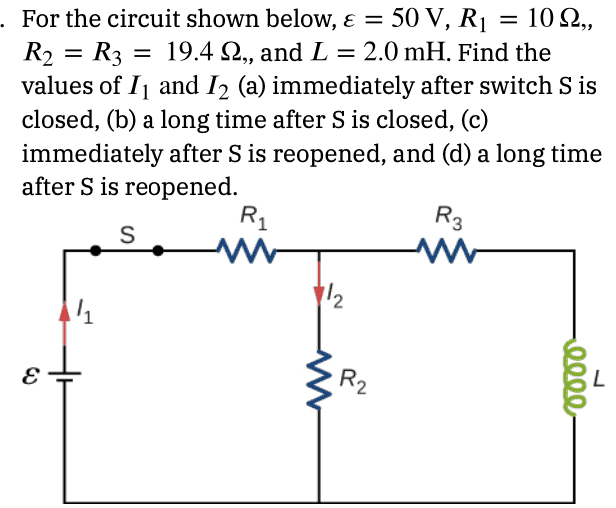
- For the circuit shown below, v = 50 V, R1 = 10 ohms, R2 = R3 = 19.4 ohms, and L = 2 mH. Find the values of I1 and I2 (c) immediately after S is reopened
- Relevant Equations
- ##\frac{LdI_3}{dt} + I_2 R_3 + I_2 R_2 = 0##
##I_2 = \frac{V}{R_3 + R_2}e^{-t/\tau}##
##I_2 = \frac{V}{R_3 + R_2}e^{-t/\tau}##
##I_2 = \frac{50}{19.4(2)}e^{-t/\tau}## as t goes to 0, ##I_2 = \frac{50}{19.4(2)}## = 1.29 Amps which is not the answer.
##I_2 = \frac{50}{19.4(2)}e^{-t/\tau}## as t goes to 0, ##I_2 = \frac{50}{19.4(2)}## = 1.29 Amps which is not the answer.
 I am always thinking of currents as positive quantities in circuit theory. I ll have to review that.
I am always thinking of currents as positive quantities in circuit theory. I ll have to review that.