Defining Characteristic Time and Distance in an Acceleration Function

[emus]

TL;DR Summary

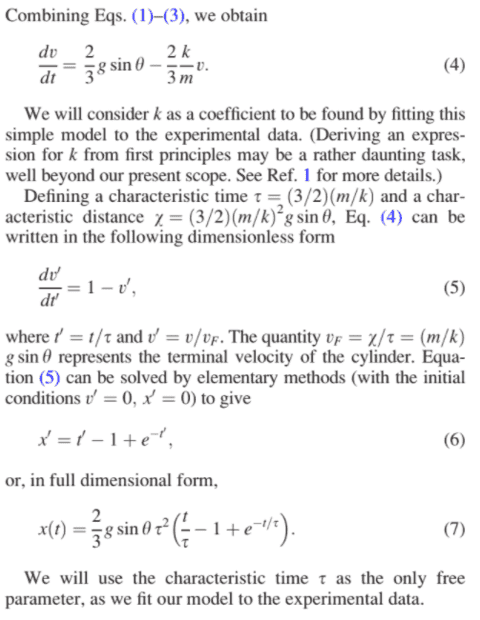
How is this equation: dv/dt = 2/3(g sinθ) - 2/3(k/m)v , nondimensionalized using characteristic time and characteristic distance?

Before starting, I will leave the link to the article I am talking about here: http://www.msc.univ-paris-diderot.fr/~phyexp/uploads/LaimantParesseux/aimant2.pdf

I am conducting a similar experiment to the one discussed in the paper above. Basically, I am rolling a neodium supermagnet down a conductive and non-conductive incline to see the effects of the eddy currents on the magnet.

If you look at "THE MODEL" section of the paper, you can see the acceleration function that is derived from Newton's equations. That is all fine and good. My problem here is, I don't know what the author means by "characteristic time" and "characteristic distance" and how the function is nondimensionalized using them.

The characteristic time is set as τ = (3/2)(m/k) and characteristic distance is set as χ = (3/2)(m/k)^2(g sinθ). I can see that these values are set by interacting with the above equation, but I don't understand exactly how and why they are used and how they are determined.

I don't really have much knowledge on the topic. I am a high school student and this topic is not in our curriculum and this is all independent learning. So, my questions are:

What is characteristic time and characteristic distance, how and why are they used?

How are the characteristic time and characteristic distance of certain equations like the one above determined?

Where does the "e" variable come from in the 5th equation and what is it??

How does the author go through with the nondimensionalization process in this case? What does he actually do?

This post may not be very clear in explaining what I am asking or what I am talking about since I am writing this at 1am and English is not my first language. If you are confused by anything I said, I would apprecaite it if you asked it in the comments below. Thanks for all the help.

PS: I don't know the level of this topic, undergrad seemed the most probable to me so I selected it. Sorry if it is incorrect.

 

[Ibix]

There are two ways to look at this. In a pure mathematics sense, the author is just neatening up a messy equation by replacing complicated products of several constants with single symbols. It's a good thing to do because you make it easier to see the mathematically important parts of the equation.

In a physics sense, the author is switching to a customised unit system where time is measured in multiples of $\tau$ and distance in multiples of $\chi$. This is undone in equation 7 which uses normal units again.

Either way, it's just a trick to make the maths neater, and it's fairly common in physics. In relativity, for example, it's standard to measure distance in units where the speed of light is 1. For example, if you measure time in years and distance in light years, speed is measured in light years per year. The physics doesn't change, but all the fiddly factors of $c$=299,792,458m/s become $c$=1 light year per year.

All you need to do to get from equation (4) to (5) is replace the messy constants with the neat ones and cancel. Can you do that?

 

[jedishrfu]

The "e" refers to the Euler number.

You can read more about its amazing properties here:

https://en.wikipedia.org/wiki/E_(mathematical_constant)

In calculus, its the one function which is its own derivative ie:

$y = e^x$ and its derivative is $dy/dx = e^x$

It also pops up in the Euler Identity, the most beautiful math expression ever:

$e^{i\pi} + 1 = 0$

where 0,1,$\pi$ and $e$ appear together.

https://en.wikipedia.org/wiki/Euler's_identity

 

[Ibix]

Oh yes - equation 5 is a differential equation. I can't teach you how to solve them in a forum post. For now, I would just accept that there are methods that let you do it.

But you can verify the solution if you can differentiate. If you calculate calculate $dx'/dt'$ and $d^2x'/dt'^2$ these are $v'$ and $dv'/dt'$. Then you can check that they satisfy (5).

 

[emus]

There are two ways to look at this. In a pure mathematics sense, the author is just neatening up a messy equation by replacing complicated products of several constants with single symbols. It's a good thing to do because you make it easier to see the mathematically important parts of the equation.

In a physics sense, the author is switching to a customised unit system where time is measured in multiples of $\tau$ and distance in multiples of $\chi$. This is undone in equation 7 which uses normal units again.

Either way, it's just a trick to make the maths neater, and it's fairly common in physics. In relativity, for example, it's standard to measure distance in units where the speed of light is 1. For example, if you measure time in years and distance in light years, speed is measured in light years per year. The physics doesn't change, but all the fiddly factors of $c$=299,792,458m/s become $c$=1 light year per year.

All you need to do to get from equation (4) to (5) is replace the messy constants with the neat ones and cancel. Can you do that?

Thanks for the reply, it cleared up a lot. I still have a few questions though.

Which "messy constants" do I replace with which "neat ones". I'm not sure since I don't see any constant to replace with time or distance. I know it is very a amateurish question but I really don't have much knowledge on the topic.

Also, how do I fit my experimental data into this formula? I have and videos of a magnet rolling down inclines of different angles. I video analyzed them and I have the position/time graphs. I also have the mass of the magnet.

I need to find the drag coefficient and I am confused about how. The author says "We will consider k as a coefficient to be found by fitting this simple model to the experimental data.". Do I simply input all my variables, calculate the acceleration using Newton's laws and find the drag coefficient? Or is there another way to independently calculate drag coefficients for this kind of motion?

Lastly, is equation (7) the function for displacement? The author uses lowercase "chi" for characteristic time and just uses x for equation (6) and (7), does he just mean displacement by that?

P.S. : I just saw your comment about differantial equations. I know how to solve simple differantial equations but I am a bit rusty, I can study and try but I just wanted to know if this is a hard one. It seems simple enough to me but do you think I can manage to solve it on my own? (with help from sources)

 

[anorlunda]

We admire your spirit in tackling such a problem while in high school.

The author says "We will consider k as a coefficient to be found by fitting this simple model to the experimental data.". Do I simply input all my variables, calculate the acceleration using Newton's laws and find the drag coefficient?

Think about the difference between experimental data and simulation data. A simulation can only reflect the equations and the parameter valued you entered. A lab experiment can reflect equations and factors beyond our knowledge or abilities.

You might consider re-running the simulation for a whole range of guessed values for the drag coefficient.

 

[emus]

We admire your spirit in tackling such a problem while in high school.Think about the difference between experimental data and simulation data. A simulation can only reflect the equations and the parameter valued you entered. A lab experiment can reflect equations and factors beyond our knowledge or abilities.

You might consider re-running the simulation for a whole range of guessed values for the drag coefficient.

Thanks for the compliment!

By "running the simulation", do you mean running the equation with the angle and mass to find the ideal acceleration? how do I make a range of guessed values for the drag coefficient? I don't have any basis to make an estimation for the drag coefficient. This is my first time dealing with an experiment like this and with drag coefficients.

If you are talking about an actual computer simulation, I don't have one. I conducted a real life experiment. Sorry, I'm just very inexperienced and couldn't find good sources to answer my questions so I'm just loading off a lot of questions here.

 

[anorlunda]

The drag coefficient is used in "the model" that you mentioned. The model is the simulation. It is used to generate predictions that are compared with experimental results.

So if the drag coefficient was dominant, you might use hill climbing optimization to find the value of drag coefficient that makes predictions best match experimental results.

But you might begin by asking yourself what you are trying to learn. If your goal is to understand the physics, then the physics apply for all values of drag coefficient.

If your goal is to learn how experiments and models are used together, you are on the right path. If that is the case, then I would begin with the simplest possible case, not such a difficult one as eddy currents and a non-uniform incline.

So perhaps you could answer the questions that any scientist would be asked by his boss, or his professor. What is your goal? How does knowing the drag coefficient relate to your goal?

 

[emus]

The drag coefficient is used in "the model" that you mentioned. The model is the simulation. It is used to generate predictions that are compared with experimental results.

So if the drag coefficient was dominant, you might use hill climbing optimization to find the value of drag coefficient that makes predictions best match experimental results.

But you might begin by asking yourself what you are trying to learn. If your goal is to understand the physics, then the physics apply for all values of drag coefficient.

If your goal is to learn how experiments and models are used together, you are on the right path. If that is the case, then I would begin with the simplest possible case, not such a difficult one as eddy currents and a non-uniform incline.

So perhaps you could answer the questions that any scientist would be asked by his boss, or his professor. What is your goal? How does knowing the drag coefficient relate to your goal?

My goal is to find the drag coefficient so I can see the strength of the induced eddy currents acting on the magnet. It is not about learning fundementals about experiments. In the end I will write a report of some sorts about the results and the experiment. It is not a school project, something extracurricular.

I admit that I am not qualified to do this. That is evident from this post itself. And I still don't understand what you mean by model. "The Model" part I mentioned was just a subsection of the paper that explained the equations that are used. I don't know how to do any of the stuff you mentioned about simulations. I don't have a computer program or any way that I know to make predictions. I feel frustrated and shame that I don't get what you are trying to mean and have to ask even more questions. All I have that has to do with this equation is:

Magnet with a mass of 13.25 grams.

The angle of the incline (this is the independent variable, range is 15-35 going up with 5 degrees)

I just need to find the drag coefficients for different angles. After thinking through again I came up with this:

Using τ=Iα and F_fr=Nμ, for a solid cylinder like I am dealing with, it can be derived that a=2/3(gsinθ).

When the effects of induced eddy currents are in place, additional drag is also in place. From the source I first shared, a = 2/3(g sinθ) - 2/3(k/m)v. [2/3(k/m)] is the effect of eddy currents on the magnet.

I think i mentioned it before, but I conducted this experiment with a conductive surface and an non-conductive surface. I have velocity-time graphs for all trials so I can calculate accelerations for all trials. For example, I calculate acceleration for the non-conductive surface at,lets say, 15°. Then I calculate acceleration at 15° for conductive surface. I find the difference between them, and that is 2/3(k/m). I have the mass, so I can find the drag coefficient for that particular trial. Than I can calculate for all trials and find the average drag coefficient for that angle.

After this is done for all angles, I can use equations to calculate the theoretical accelerations and drag coefficients, compare them in the conclusion section and see if the theoretical values are in the uncertainty range of the experimental values and evaluate the experiment as a whole. I think that would make the most compelling report I can with the current situation.

I am not demanding direct answers from you or anyone, but as more experienced and qualified physicists, I hope you can at least give your opinions on my ideas. Thanks for all your time and effort.

 

[anorlunda]

There is no need to be embarassed. No matter how much you learn, there is still much more you don't know. None of us can learn everything.

This quote is from the paper you linked in post #1.

We will consider k as a coefficient to be found by fitting this simple model to the experimental data. (Deriving an expression for k from first principles may be a rather daunting task, well beyond our present scope. See Ref. 1 for more details.)

So they found k by "fitting." That means using a computer to find a curve that best "fits" the experimental data. A method called "least squares fitting" is used for that.

I need to find the drag coefficient and I am confused about how. The author says "We will consider k as a coefficient to be found by fitting this simple model to the experimental data.". Do I simply input all my variables, calculate the acceleration using Newton's laws and find the drag coefficient? Or is there another way to independently calculate drag coefficients for this kind of motion?

You should know the acceleration from the experiments. Velocity is the rate of change of distance with time, and acceleration is the rate of change of velocity with time. So if you have the data for position at every time point, you can calculate velocity and accleration from that.

The equation in the paper is: $mg\sin \theta - f - kv = ma$

It looks to me like there are two unknown factors f for friction and kv for drag. But if you know all the other terms, including acceleration, you can solve the equation for $(f - kv)$.

Other PF members who help students with physics experiments may be able to advise you better.

 

[emus]

There is no need to be embarassed. No matter how much you learn, there is still much more you don't know. None of us can learn everything.

This quote is from the paper you linked in post #1.So they found k by "fitting." That means using a computer to find a curve that best "fits" the experimental data. A method called "least squares fitting" is used for that.You should know the acceleration from the experiments. Velocity is the rate of change of distance with time, and acceleration is the rate of change of velocity with time. So if you have the data for position at every time point, you can calculate velocity and accleration from that.

The equation in the paper is: $mg\sin \theta - f - kv = ma$

It looks to me like there are two unknown factors f for friction and kv for drag. But if you know all the other terms, including acceleration, you can solve the equation for $(f - kv)$.

Other PF members who help students with physics experiments may be able to advise you better.

Thanks!

I thought fitting meant just inserting the experimental values into the equation and solving for "k". I will look into "least squares fitting".

As for solving for $(f-kv)$, from equation (2) in the paper I linked, you can find $f = Iα/R$. You can then insert moment of inertia for solid cylinder and substitute $a/R$ for α.

And then you can substitute that f value into $mg\sin \theta - f - kv = ma$, you can simplify the formula into $a = 2/3(g\sinθ) - (2/3)(k/m)v$ and I only have to solve for k. For that I think the method I mentioned might work. I will try it. Thanks for your comments.