Definition of Clifford Algebras

[jv07cs]

I was reading D.J.H. Garling's book "Clifford Algebra: An Introduction" and it defines clifford algebras as follows:

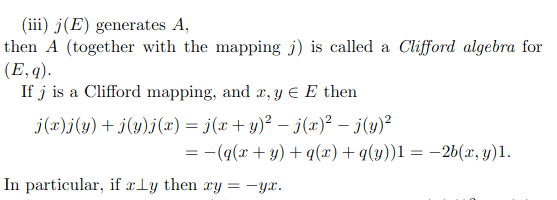
But if $1 \notin j(E)$, how come $j(E)$ generate $A$ since it doesn't generate its identity element?

 

[Office_Shredder]

You could have elements 1+x and -x in the range of j, and then 1 is contained in the algebra generated by the range

 

[jv07cs]

You could have elements 1+x and -x in the range of j, and then 1 is contained in the algebra generated by the range

But if, let's say, $j(v) = 1 + x$ and $j(w) = -x$, for $v,w \in E$. The element $u = v + w$ is also in $E$ and since $j$ is linear, we would have $j(u) = j(v+w) = j(v) + j(w) = 1$, which would imply $1 \in j(E)$, right?

 

[Office_Shredder]

But if, let's say, $j(v) = 1 + x$ and $j(w) = -x$, for $v,w \in E$. The element $u = v + w$ is also in $E$ and since $j$ is linear, we would have $j(u) = j(v+w) = j(v) + j(w) = 1$, which would imply $1 \in j(E)$, right?

Sorry you're right you need multiplication to make things interesting. E.g. you could have some $x,y$ in the range of $j$ such that $xy=1$ (or maybe you need to layer some addition on top of multiplication, I haven't thought carefully about it obviously)

 

[jv07cs]

Sorry you're right you need multiplication to make things interesting. E.g. you could have some $x,y$ in the range of $j$ such that $xy=1$ (or maybe you need to layer some addition on top of multiplication, I haven't thought carefully about it obviously)

Oh okay. So by "$j$ generates $A$" we don't limit ourselves to just linear combinations, we should also consider the algebra multiplication right?

 

[martinbn]

Generates as an algebra over the given field means the smalest algebra that contains the gwnerating set. It will also contain the field. The same way that the set of one element $x$ generates the algebra of polynomials in $x$.

 

[Office_Shredder]

Oh okay. So by "$j$ generates $A$" we don't limit ourselves to just linear combinations, we should also consider the algebra multiplication right?

This is correct

 

[nuuskur]

When you say $j(E)$ generates $A$, which is an algebra, then it's already implicit that one considers the smallest subalgebra that contains $j(E)$. IOW, it's the set of all linear combinations of finite products of the generating set - polynomials, if you will.