Definition of first order infinitesimal using equivalence class

[Mike_bb]

Hello!

I'm studying Synthetic Differential Geometry and I read about model construction of SDG.
I found such source: http://www.iam.fmph.uniba.sk/amuc/_vol-73/_no_2/_giordano/giordano.pdf

I have questions about extension R to *R and about definition of first order infinitesimal using equivalence classes.
1.) I can't understand how "the class generated by h(t)=t could be a first order infinitesimal number" (see below). How is it possible? How does it work? What is idea of this?

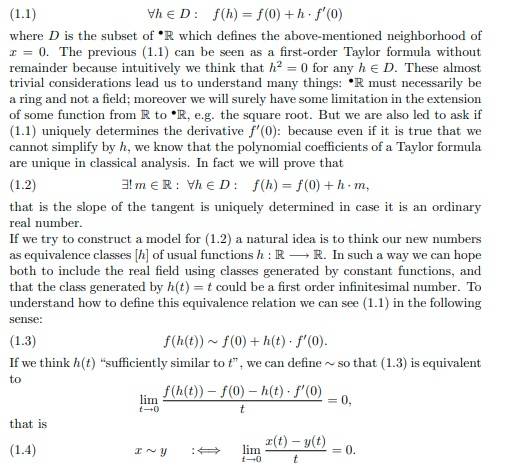
2.) How is it possible to define D using the condition of limsup? How did author prove that D is an ideal of *R using properties of limsup? I can't understand it.

Thanks!!

 

[Office_Shredder]

To prove its an ideal of a ring you need two things. It's closed under addition, and multiplying an element of the ideal by any element of the ring gives an element of the ideal. h and k are ideal elements and x is an arbitrary ring element in the proof

 

[Mike_bb]

To prove its an ideal of a ring you need two things. It's closed under addition, and multiplying an element of the ideal by any element of the ring gives an element of the ideal. h and k are ideal elements and x is an arbitrary ring element in the proof

Thanks. What do you think about "the class generated by h(t)=t could be a first order infinitesimal number"? Does it mean that h(t)=t is infinitesimal function (t->0)?

 

[Office_Shredder]

Really it just means $h(t)=t$ is an element of $D$. They're just trying to describe in words the math that is about to come