Deflection of partially loaded cantilever beam with non-homogeneous EI

[elepolli]

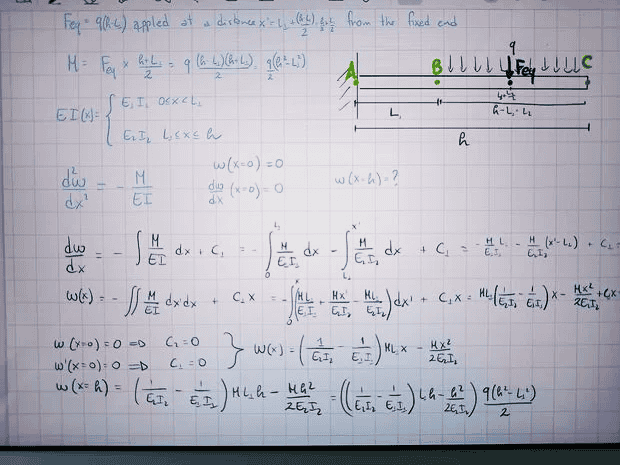
TL;DR Summary: I have a cantilever beam with fixed end, known rectangular cross section and total length h. A uniform load is applied on the beam from a distance L from the fixed end, to the free end. The E modulus and inertia I are known, and they are two different constant values for 0<x<L and L<x<0.
I want to know the deflection w(h) of the beam at the free end.

This is my approach, what do you think?

 

[erobz]

Your image is loaded sideways.

For $0 < x_1 \leq L_1$ you solve

$$ \frac{d^2y}{dx_1^2} = \frac{M_1(x_1)}{E_1I_1} $$

Then, for $L_1 < x_2 \leq L_2$ you can solve

$$ \frac{d^2y}{dx_2^2} = \frac{M_2(x_2)}{E_2I_2} $$

Where the constants of integration (slope, deflection) at $x_2 = 0$ come from the end condition of the first section.

 

[elepolli]

Your image is loaded sideways.

For $0 < x_1 \leq L_1$ you solve

$$ \frac{d^2y}{dx_1^2} = \frac{M_1(x_1)}{E_1I_1} $$

Then, for $L_1 < x_2 \leq L_2$ you can solve

$$ \frac{d^2y}{dx_2^2} = \frac{M_2(x_2)}{E_2I_2} $$

Where the constants of integration (slope, deflection) at $x_2 = 0$ come from the end condition of the first section.

How should I calculate $M_1$ and $M_2$?

 

[erobz]

How should I calculate $M_1$ and $M_2$?

Find the reactions at point A( force and moment). Then you write the internal moment at the end of the section as function of x in the typical way(by inspection, or integrating the shear).