Derivative of a Function: Understanding Slope and Continuity

[grace77]

Problem statement

My question is for number 27.
Revelant equation
None

Attempt at a solution

I'm not sure where to start.

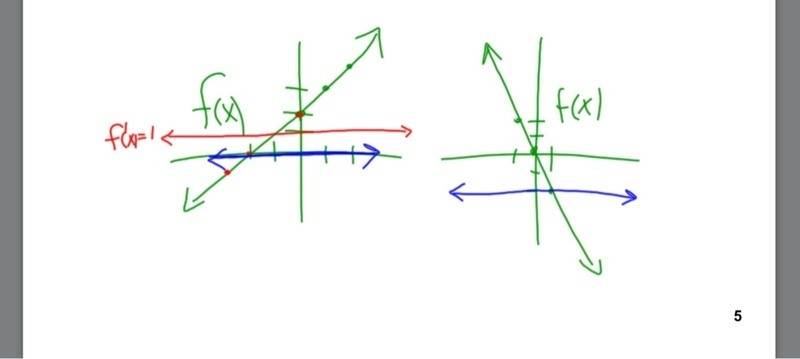
This is my teachers answer. I understand how the slope is 1 for x greater than -1 and that it is -2 at x greater than -1 and that there is a point at (0,-1) but I don't understand how they connect to form that final pic. I think I'm missing something ,can someone help me?

 

[grace77]

Never mind I think I understand it now. I think I was just confused by the previous picture leading up to the answer.

 

[grace77]

Never mind I think I understand it now. I think I was just confused by the previous picture leading up to the answer.

Maybe someone could elaborate on this for me? Thanks

 

[SammyS]

Maybe someone could elaborate on this for me? Thanks

What does it mean for a function to be continuous ?

 

[grace77]

What does it mean for a function to be continuous ?

That there are no breaks or holes

 

[HallsofIvy]

If f'(x)= 1, for x< -1, then f(x)= x+ C1 for some constant C1, for x< -1, so the graph is a straight line with slope 1.

If f'(x)= -2, for x> -1, then f(x)= -2x+ C2 for some constant C2, for x> -1, so the graph is a straight line with slope -2.

Since f is continuous, the two lines must meet at x= -1. That means that -1+ C1= -2(-1)+ C2.

That, together with f(0)= C2= -1 is sufficient to determine both C2 and C1.