Hi
@jordy1113 : this is an interesting question! I think it might be useful to step back for a minute and see where all this is coming from, rather than just blindly substituting one equation into another. For
any constituent of the "perfect" (largely isotropic & homogenous) "fluid" that we consider to be permeating the Universe, we can write its equation of state as
$$P = w \rho $$
Where ##P## is the pressure of this fluid constituent, and ##\rho## is its density (if we take ##c = 1##, then ##\rho## is the energy density). Again, these quantities could describe any individual constituent, whether it be, matter, radiation, or dark energy. Now consider at time ##t##, (when the scale factor was ##a(t)##),
any finite volume ##V## in the Universe. It has internal energy (due to this constituent) of ##U = \rho V##. We can consider it to be expanding adiabatically*, so that
$$dU = -P dV $$
Using just the above two equations, you can derive the following first-order differential equation for ##\rho(a)##, which is the variation of our constituent's energy density with scale factor.
I strongly encourage you to derive this ODE for yourself. Hint: from the definition of the scale factor, the volume is just given by: ##V = V_0a^3##, where ##V_0## is the volume of our region now (at the present day).
*Note that the "adiabatic expansion" argument is a bit hokey, but it does give the right answer. A more rigorous GR-like way to derive this ODE is to consider that the "covariant derivative" of the stress-energy tensor must equal 0, for a perfect fluid. See e.g. equation 2.55 of
Modern Cosmology by Scott Dodelson. Anyway, the ODE IS
$$\frac{d\rho}{da} + \frac{3}{a}\rho(1+w) = 0 $$
You can solve this ODE by method of integrating factors (or whatever) to find that the variation of density with scale factor for
any constituent is given by:
$$\boxed{\rho(a)=\rho_0a^{-3(1+w)}}$$
where ##\rho_0 = \rho(a=1)## is the density at the present day.
For matter (baryonic or dark), ##w = 0##, giving us a ##\rho_m(a) = \rho_m^0 a^{-3}## dependence as expected, since the number density of matter particles in any given volume just dilutes with the expansion. Here ##\rho^0_m## is just the present-day matter density, and I've just shifted the "naught" from subscript to superscript (like in your notes above) to make room for a subscript telling you what constituent we're dealing with.
For radiation (specifically for an isotropic blackbody radiation field), it can be shown that the relation between radiation pressure and energy density is given by ##w = 1/3##, leading to a ##\rho_r = \rho_r^0a^{-4}## dependence: again this as expected, because number density of photons in a given volume dilutes as ##a^{-3}##, but then energy
per photon decreases by a further factor ##a^{-1}## due to increase of photon wavelength linearly with ##a## (cosmological redshift).
For the simplest form of dark energy, equivalent to a cosmological constant, we take ##w = -1##, which from the boxed equation above, clearly leads to ##\rho_{d}(a) = \rho^0_d = \mathrm{const}##. However, the whole point of your exercise is to allow for the
possibility of more complicated time-dependence of the dark energy density, since the exact time dependence is unknown right now (we need more observational data to constrain it). We introduce this time-dependence as a scale-factor dependence of the equation-of-state parameter for dark energy: ##w = w(a) = w_0 + ## (some function of ##a##). To be honest, I would assume that ##w_0 = -1## here, since the whole point of this parameterization of ##w## is to separate out its time-dependent and time-independent components. Anyway, for now, let's just keep ##w## (for dark energy) fully general and see how far we can get without specifying a functional form for it. Again from the boxed equation:
$$\rho_d(a)=\rho_d^0a^{-3(1+w)}$$
By
definition, the density parameter ##\Omega_X## for constituent ##X## is just the ratio of this constituent's energy density to the critical density. Therefore:
$$\Omega_d(a) \equiv \frac{\rho_d(a)}{\rho_\mathrm{crit}(a)} = \frac{\rho_d^0a^{-3(1+w)}}{\rho_\mathrm{crit}(a)}$$
But what is ##\rho_\mathrm{crit}##, and how does it vary with ##a##? Well, take a look at the
First Friedmann Equation. We know that if the total density ##\rho_\mathrm{tot}## of all constituents is equal to the critical density, then the Universe will be geometrically flat (Euclidean). We can therefore set the
curvature term in the First Friedman Equation to zero, just giving us:
$$H^2 = \frac{8\pi G}{3}\rho_\mathrm{tot} = \frac{8\pi G}{3}\rho_\mathrm{crit}$$
From this it follows that at any time in the Universe's history when the scale factor was ##a##:
$$\rho_\mathrm{crit} = \frac{3H^2}{8\pi G}$$
And of course, evaluating the Friedmann equation at the present day:
$$\rho^0_\mathrm{crit} = \frac{3H_0^2}{8\pi G}$$
Therefore:
$$\frac{\rho_\mathrm{crit}}{\rho^0_\mathrm{crit}} = \frac{H^2}{H_0^2}$$
As a trick, I figured we could substitute this into the ##\Omega_d## equation above:
$$\Omega_d(a) = \frac{\rho_d^0a^{-3(1+w)}}{\rho^0_\mathrm{crit}\frac{\rho_\mathrm{crit}}{\rho^0_\mathrm{crit}}} = \frac{\Omega_d^0a^{-3(1+w)}}{\frac{\rho_\mathrm{crit}}{\rho^0_\mathrm{crit}}} = \frac{\Omega_d^0a^{-3(1+w)}}{\left(\frac{H}{H_0}\right)^2}$$
This last equality is useful, because the denominator is just the First Friedmann Equation (lefthand side) again, albeit written in a slightly different form:
$$\left(\frac{H}{H_0}\right)^2 = \Omega_r^0a^{-4} + \Omega_m^0a^{-3} + \Omega_k^0a^{-2} + \Omega_da^{-3(1+w)}$$
Again,
I strongly encourage you to derive this version of the First Friedmann Equation for yourself. You have all the info you need to above. Hint: bear in mind that ##\rho_\mathrm{tot} = \rho_r + \rho_m + \rho_d##
Anyway, we can substitute this righthand side into the denominator of our Omega_d equation to produce:
$$\boxed{\Omega_d(a) = \frac{\Omega_d^0a^{-3(1+w)}}{\Omega_r^0a^{-4} + \Omega_m^0a^{-3} + \Omega_k^0a^{-2} + \Omega_da^{-3(1+w)}}}$$
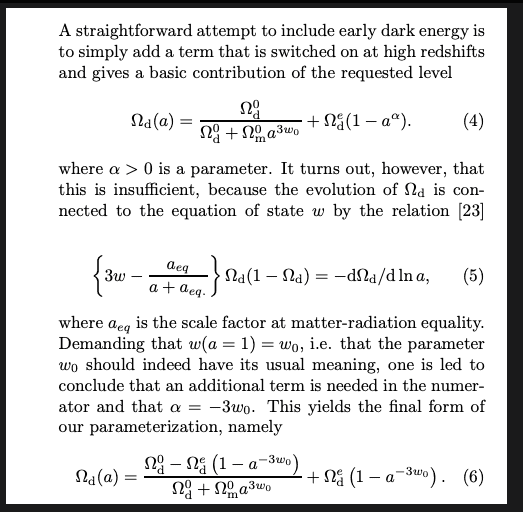
Now we're getting somewhere! What I mean by that is that I seem to be edging closer to something that looks like your equations 4 and 6, but in a way that is fully general, derived from first principles, without any gobbledygook.
A Few Questions
- Can you consider the Universe to be flat for the purposes of this exercise? I.e. can you set the curvature density parameter ##\Omega_k^0 = 0##?
- What form exactly is your prof using to parameterize ##w(a)##? Is it something like ##w = w_0 + \alpha/a##, where alpha is an ad hoc parameter to be fitted to the data? Whatever it is, it would need to be substituted in everywhere you see ##w## above.
- Where the heck does the Epoch of Matter-Radiation Equality come in? I think I can see a possible benefit to using it as a reference epoch where the Friedmann equations take a similar form. After all, presumably the whole point of considering the time of equality would be that ##\Omega_r(a_\mathrm{eq}) = \Omega_m(a_\mathrm{eq})##, potentially allowing for some simplifications in the last boxed equation above.
If I had more time to play around with this, then what I said in point 3 would be my next direction to take. I.e. I might play around with ratios like ##\Omega_d(a) / \Omega_d(a_\mathrm{eq}) ##
But unfortunately I don't have any more time. Can you take it further from here?