Deriving general specific heat capacity formula

[member 731016]

Homework Statement

Please see below

Relevant Equations

Please see below

For this,

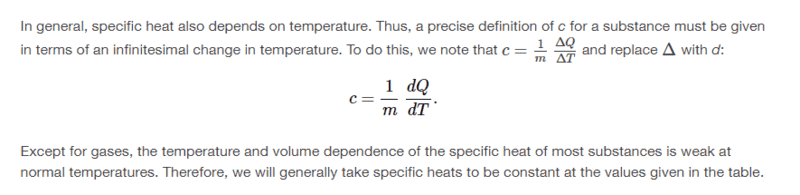
Dose anybody please know of a better way to derive the formula without having $c = \frac{\Delta Q}{m \Delta T}$ then taking the limit of both sides at $\Delta T$ approaches zero? I thought $\Delta Q$ like $\Delta W$ was not physically meaningful since by definition $Q$ is the heat transfer.

Many thanks!

 

[kuruman]

I thought $\Delta Q$ like $\Delta W$ was not physically meaningful since by definition $Q$ is the heat transfer.

Do you think that $Q$ is physically meaningful?

 

[member 731016]

Do you think that $Q$ is physically meaningful?

Thank you for your reply @kuruman!

Yes I do, since it is the quantity of heat transferred.

Many thanks!

 

[kuruman]

Thank you for your reply @kuruman!

Yes I do, since it is the quantity of heat transferred.

Many thanks!

Well, $Q$, which is physically meaningful, is not transferred instantaneously all at once but in increments $\Delta Q$. Why is $Q$ meaningful but not an element $\Delta Q$ that is part of it? BTW, the same reasoning applies to $\Delta W.$

 

[member 731016]

Well, $Q$, which is physically meaningful, is not transferred instantaneously all at once but in increments $\Delta Q$. Why is $Q$ meaningful but not an element $\Delta Q$ that is part of it? BTW, the same reasoning applies to $\Delta W.$

Thank you for your reply @kuruman!

So could we think of the heat transferred as the summation of the differential heat elements $dQ$ which I think leads to $Q = \int dQ$.

However, back to the algebra way of thinking, is the reason why the heat element $\Delta Q$ is not meaningful because it is causes a differential change in the state of the system that can be considered negligible?

Many thanks!

 

[kuruman]

Thank you for your reply @kuruman!

So could we think of the heat transferred as the summation of the differential heat elements $dQ$ which I think leads to $Q = \int dQ$.

However, back to the algebra way of thinking, is the reason why the heat element $\Delta Q$ is not meaningful because it is causes a differential change in the state of the system that can be considered negligible?

Many thanks!

Why do you insist $\Delta Q$ is not meaningful? In post #3 you agreed that it is.

 

[member 731016]

Why do you insist $\Delta Q$ is not meaningful? In post #3 you agreed that it is.

Thank you for your reply @kuruman!

Yeah I guess it is meaningful if we think of it has a differential heat element not as $Q_f - Q_i$ which cannot be true since heat is state variable.

Many thanks!

 

[nasu]

The specific heats are defined in terms of derivatives of intenal energy or entropy not of heat. And as definitions, they cannot be proven.
The heat is not a function of state so using the derivative of heat in respect to temperature it may be a little hand waving.

 

[member 731016]

The specific heats are defined in terms of derivatives of intenal energy or entropy not of heat. And as definitions, they cannot be proven.
The heat is not a function of state so using the derivative of heat in respect to temperature it may be a little hand waving.

Thank you for your reply @nasu!

That is very interesting what you mention. Sorry I did quite get the bit I put it italic above. I don't understand the bit about hand waving. Is it still correct what the textbook did?

Many thanks!