theshape89
- 5
- 2
- Homework Statement
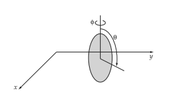
- A disk rolls without slipping across a horizontal plane. The plane of the disk remains vertical, but it is free to rotate about a vertical axis. What generalized coordinates may be used to describe the motion? Write a differential equation describing the rolling constraint. I've attached a picture below.
- Relevant Equations
- Let ##\omega## be the angular velocity of a point on the edge of the disk and ##r## the radius of the disk. Then ##v_{tangential}=\omega r##.
Let ##R=\sqrt{x^{2} + y^{2}}##. Then
\begin{align}v_{tangential}&=\frac{dR}{dt} \nonumber\\
&=\frac{dR}{dx}\frac{dy}{dt} + \frac{dR}{dy}\frac{dy}{dt} \nonumber\\
&=\frac{x}{R}\frac{dx}{dt} + \frac{y}{R}\frac{dy}{dt} \nonumber\\
&= cos\phi \frac{dx}{dt} + sin\phi \frac{dy}{dt}.\nonumber \end{align}
Using ##v_{tangential}=\omega r##
I'm supposed to end up with
\begin{align}cos\phi dx+ sin\phi dy &= d\theta r\end{align}
and
\begin{align}\frac{dy}{dx}=tan\phi\end{align}.
But I'm not sure how to get (2). If I differentiate (1) w.r.t. ##x## and if the terms with ##\frac{d\phi}{dx}## disappear, I'm still left with ##cos\phi + sin\phi \frac{dy}{dx}=0##, which won't get me to (2).
\begin{align}v_{tangential}&=\frac{dR}{dt} \nonumber\\
&=\frac{dR}{dx}\frac{dy}{dt} + \frac{dR}{dy}\frac{dy}{dt} \nonumber\\
&=\frac{x}{R}\frac{dx}{dt} + \frac{y}{R}\frac{dy}{dt} \nonumber\\
&= cos\phi \frac{dx}{dt} + sin\phi \frac{dy}{dt}.\nonumber \end{align}
Using ##v_{tangential}=\omega r##
##cos\phi \frac{dx}{dt} + sin\phi \frac{dy}{dt} = \frac{d\theta}{dt}r##
or##cos\phi dx+ sin\phi dy= d\theta r##
I'm supposed to end up with
\begin{align}cos\phi dx+ sin\phi dy &= d\theta r\end{align}
and
\begin{align}\frac{dy}{dx}=tan\phi\end{align}.
But I'm not sure how to get (2). If I differentiate (1) w.r.t. ##x## and if the terms with ##\frac{d\phi}{dx}## disappear, I'm still left with ##cos\phi + sin\phi \frac{dy}{dx}=0##, which won't get me to (2).