Detailed solution of an envelopes example in a math book

[vgarg]

TL;DR Summary

I am looking for steps for the solution of an envelopes example in Mathematical Methods for Physics and Engineering book

Can someone please show me the steps from circled equation 1 to 2?

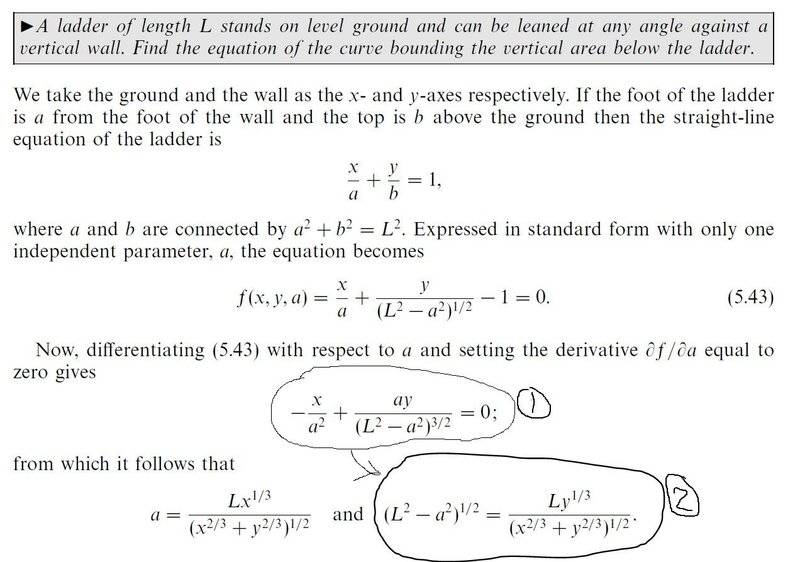
Thank you.

 

[Hill]

If you replace $x \leftrightarrow y$ and $a \leftrightarrow b$ in the previous equation, you get the last equation.

 

[erobz]

I believe the author of your text book? @Orodruin

 

[Orodruin]

I believe the author of your text book? @Orodruin

No. There are several textbooks with that or similar names. This is from Riley et al. I believe.

@vgarg This is why you should never just quote the name of the book, but also the author(s).

 

[DrClaude]

If you replace $x \leftrightarrow y$ and $a \leftrightarrow b$ in the previous equation, you get the last equation.

To clarify: By the symmetry of the problem, you could have solved it for $b$ to get
$$
b = \frac{L y^{1/3}}{(x^{2/3} + y^{2/3})^{1/2}}
$$
instead of
$$
a = \frac{L x^{1/3}}{(x^{2/3} + y^{2/3})^{1/2}}
$$

Then simply substitute $b$ in the first equation using $a^2 + b^2 = L^2$.

 

[vgarg]

Thank you very much for your help. I will sure list the author of the book in any future post.