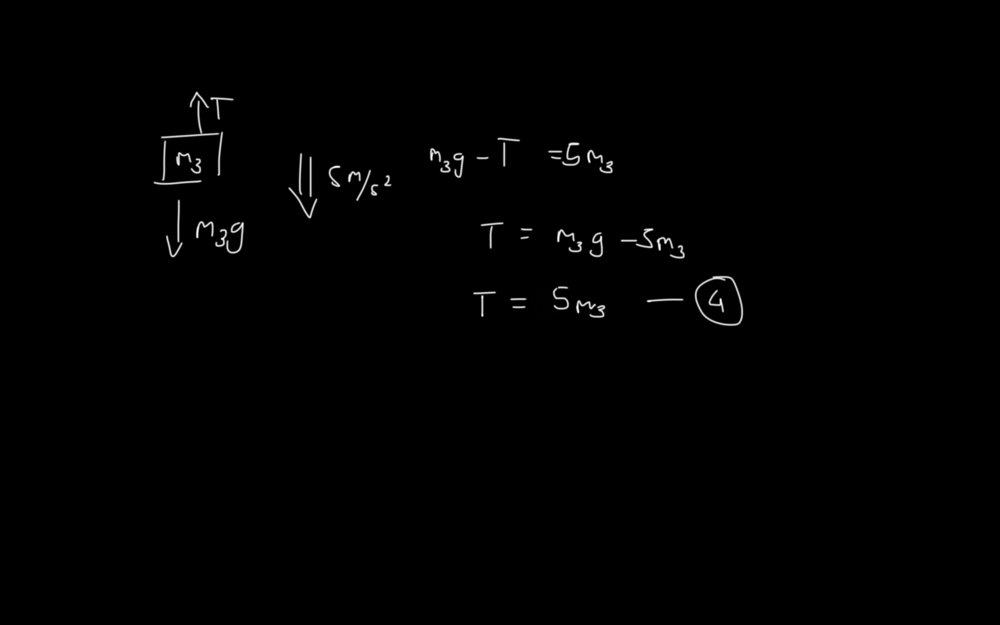Determine the acceleration of block m1 in this wedge-pulley system

[Kaushik]

Homework Statement

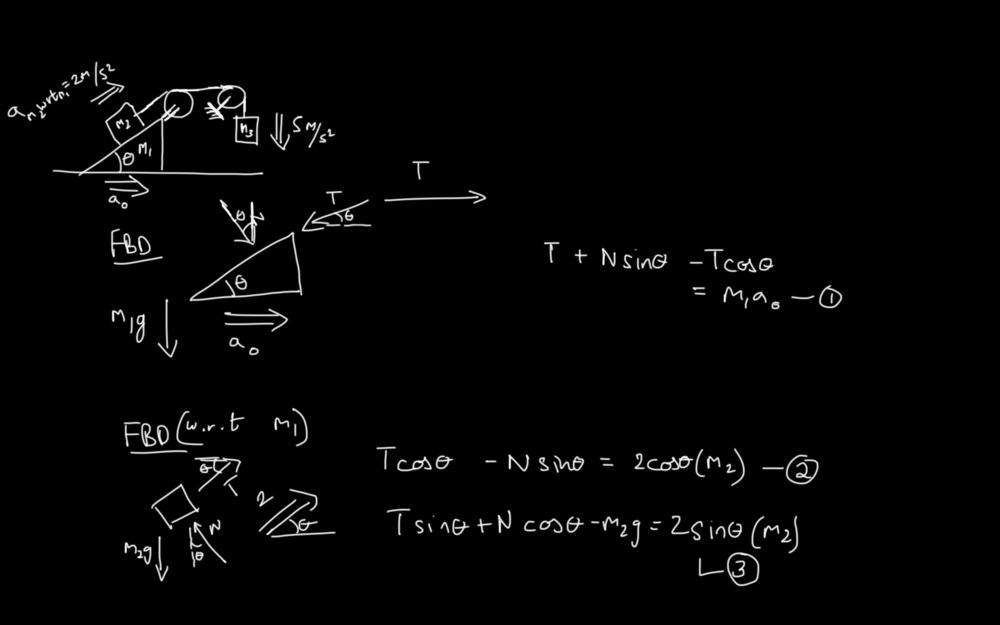
The acceleration of block m2 with respect to m1 is 2m/s^2 upward along the inclination. The block m3 accelerates downward with 5 m/s^2.

Relevant Equations

a( of m2 wrt m1) = 2m/s2
a(of m3) = 5m/s2

The equations i got are attached below. Is it right? If yes what should we do after this. I tried solving the equations, but i did not arrive at the solution.

 

[jbriggs444]

What quantity are you trying to determine? If it is $a_0$ (the rightward acceleration of block $m_1$) then you seem to be overthinking it.

 

[Kaushik]

What quantity are you trying to determine? If it is $a_0$ (the rightward acceleration of block $m_1$) then you seem to be overthinking it.

Yes. I am trying to determine $a_0$. So, do we have an easy way to solve this problem?

 

[jbriggs444]

Yes. I am trying to determine $a_0$. So, do we have an easy way to solve this problem?

Yes.

Let us start with a simpler version of the problem. If blocks $m_1$ and $m_2$ were glued together, how rapidly would the pair need to be accelerating rightward so that $m_3$ would be accelerating downward at 5 m/sec²?

 

[Kaushik]

Yes.

Let us start with a simpler version of the problem. If blocks $m_1$ and $m_2$ were glued together, how rapidly would the pair need to be accelerating rightward so that $m_3$ would be accelerating downward at 5 m/sec²?

$5 \frac{m}{s^2}$?

 

[jbriggs444]

$5 \frac{m}{s^2}$?

Yes indeed.

If block $m_1$ were glued to the ground while block $m_2$ were once again free to slide, how rapidly would it need to be accelerating so that block $m_3$ would be accelerating downward at 5 m/sec²?

 

[Kaushik]

Yes indeed.

If block $m_1$ were glued to the ground while block $m_2$ were once again free to slide, how rapidly would it need to be accelerating so that block $m_3$ would be accelerating downward at 5 m/sec²?

$5 \frac{m}{s^2}$again? as the pulley attached to $m_1$ is not moving anymore?

 

[jbriggs444]

$5 \frac{m}{s^2}$again? as the pulley attached to $m_1$ is not moving anymore?

Yes. Now unglue all blocks and return to the original problem. Can you write an equation for the downward acceleration of $m_3$ in terms of the rightward acceleration of $m_1$ and the diagonal acceleration of $m_2$?

[If it were me, I would label the accelerations of $m_1$, $m_2$ and $m_3$ as $a_1$, $a_2$ and $a_3$]

 

[Kaushik]

Yes. Now unglue all blocks and return to the original problem. Can you write an equation for the downward acceleration of $m_3$ in terms of the rightward acceleration of $m_1$ and the diagonal acceleration of $m_2$?

[If it were me, I would label the accelerations of $m_1$, $m_2$ and $m_3$ as $a_1$, $a_2$ and $a_3$]

Is $a_0 = 3 \frac{m}{s^2}$?

 

[jbriggs444]

Is $a_0 = 3 \frac{m}{s^2}$?

Yes

 

[Kaushik]

Yes

Oh, thanks. But in my book the solution given was 2. So is the solution given in my book wrong?

 

[jbriggs444]

Oh, thanks. But in my book the solution given was 2. So is the solution given in my book wrong?

Yes, assuming the question is as stated then the book is wrong.

 

[Kaushik]

Yes

I used relative acceleration.

If the block $m_3$ is accelerating downwards with 5, then string that is horizontal should also accelerate right with 5. But the acceleration of that horizontal string with respect to the block $m_1$ should be 2.

Let the horizontal string be h.

$a_h = 5 \frac{m}{s^2}$
$a_{h}$ ( with respect to $m_1$ ) $= 5 - a_0$

But as $a_h$(with respect to $m_1$ ) $= 2 \frac{m}{s^2}$

we get $a_0 = 3 \frac{m}{s^2}$

 

[Kaushik]

Yes, assuming the question is as stated then the book is wrong.

Thanks for your help !