- #1
dykuma
- 56
- 7
- Homework Statement
- An electromagnetic wave is incident into the flat surface of an unknown material. In the surface, the transverse wave mode follows the relation ##\omega^2 = \omega_m + c^2 k^2## , where ##\omega_m## is an angular frequency associated with the material. The angular frequency of the incident wave is ##\omega = 2 \omega_m##
What is the incident wavelength and the transverse wavelength in terms of the speed of light and the angular frequency associated with the material? Then, determine the critical angle such that there is no transmission into the material?
- Relevant Equations
- I'm expecting to use snell's law, as well as equations related to wave numbers and the index's of refraction.
First, I attempted to find the wavelength of the incident wave in the required terms:

Next, I tried to find the wavelength inside of the material:
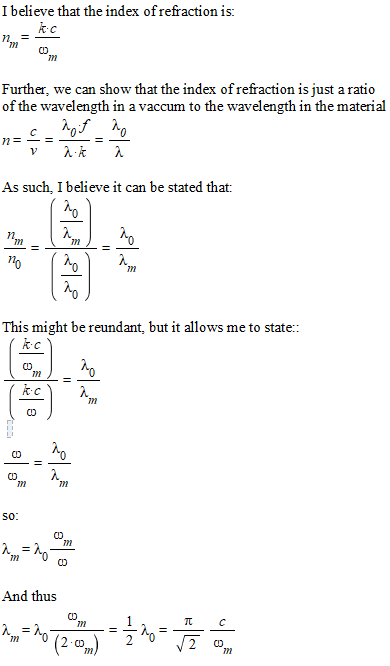
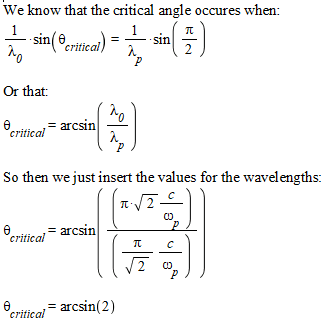
And then lasted, I tried to find the critical angle:
My issue is that
1) I don't know if what I did was correct.
2) I don't think what I did was correct because that angle is in the complex plane. Maybe this unknown material is wild stuff, but I'm not sure that is possible. If I invert the answer, (that is, assume that I did the problem backwards and mixed up the wavelengths),I get a critical angle of pi/6, which is almost too nice not to be the right answer.
Anyway, any help or direction on this problem would be appreciated.
Next, I tried to find the wavelength inside of the material:
And then lasted, I tried to find the critical angle:
My issue is that
1) I don't know if what I did was correct.
2) I don't think what I did was correct because that angle is in the complex plane. Maybe this unknown material is wild stuff, but I'm not sure that is possible. If I invert the answer, (that is, assume that I did the problem backwards and mixed up the wavelengths),I get a critical angle of pi/6, which is almost too nice not to be the right answer.
Anyway, any help or direction on this problem would be appreciated.
