Determine the linear acceleration of the two masses

[Crystal]

Homework Statement

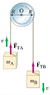
Two masses, mA = 40.0 kg and mB = 55.0 kg, are connected by a massless cord that passes
over a pulley that is free to rotate about a fixed axis. This device is known as an
Atwood’s Machine. The pulley is a solid cylinder of radius R = 0.360 m and mass of 7.50
kg.
1. Determine the linear acceleration of the two masses
2. Determine the angular acceleration of the pulley

Homework Equations

a=atan+ar

The Attempt at a Solution

not sure how to start, any hints would be great

 

[Satvik Pandey]

Welcome to PF:)
First draw free body diagrams of $m_{a}$,$m_{B}$ and pulley separately.
Try to make equations by using Newton's second law of motion and rotation.

 

[Crystal]

Welcome to PF:)
First draw free body diagrams of $m_{a}$,$m_{B}$ and pulley separately.
Try to make equations by using Newton's second law of motion and rotation.

for Ma I got 9.8*40=392
Mb 9.8*55=539
making the total force is 147.

I also know that to find net torque of the pulley its =mratan.

What should I attempt next?

 

[NTW]

There's a way to solve the problem 'by energies', equating the PE of the system with its total KE 'after the fall', giving that fall some arbitrary value. You should remember that the total KE of this system is the sum of its linear kinetic energy and its rotating kinetic energy...

 

[Satvik Pandey]

for Ma I got 9.8*40=392
Mb 9.8*55=539
making the total force is 147.

I also know that to find net torque of the pulley its =mratan.

What should I attempt next?

I have made free body diagrams for you

Can you form equations using Newton's second law of motion and rotation.
Just use variables as shown in diagram. Do not put the numerical value right now.

 

[Crystal]

so

fnet = total mass * acceleration
fnet = the sum of all forces on both objects
making it
total mass * linear acceleration = sum of all forces

linear acceleration = 9.8(-40+55+7.5)/(40+55+7.5)
linear acceleration = 2.15rad/s^2

 

[NTW]

The pulley does not fall not rise or fall, and its mass should not be added to the other two masses. And its weight plays no role...

The contribution of the pulley is to decelerate the masses absorbing a part of the variation of their PE in the form of rotational KE. That's why I pointed out that the problem could be solved 'by energies', equating the loss of PE of the system with the increase of linear KE plus rotational KE...

 

[Crystal]

The pulley does not fall not rise or fall, and its mass should not be added to the other two masses. And its weight plays no role...

The contribution of the pulley is to decelerate the masses absorbing a part of the variation of their PE in the form of rotational KE. That's why I pointed out that the problem could be solved 'by energies', equating the loss of PE of the system with the increase of linear KE plus rotational KE...

so use the formula 1/2mv^2+1/2Iw^2?