Determine the ratio ##OA:AR## in the vector problem

[chwala]

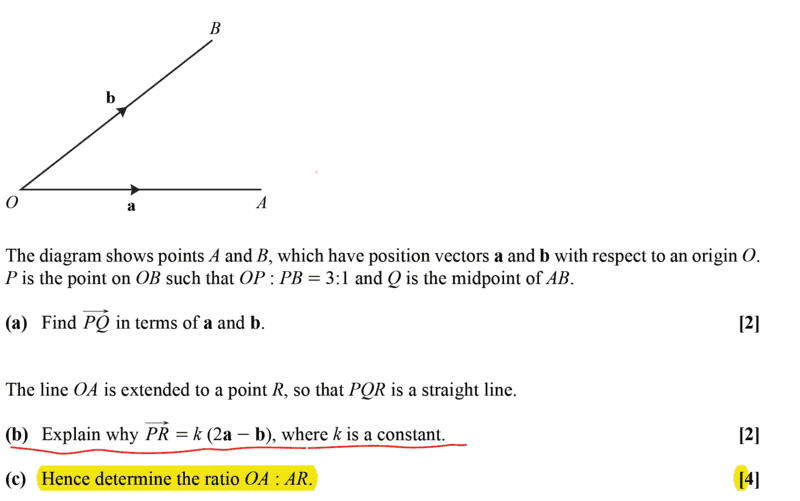
Homework Statement

see attached

Relevant Equations

Vectors

My question is on part (c) only.

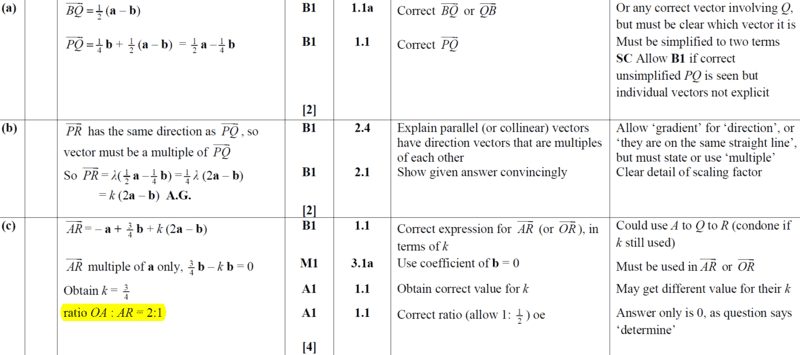
Find the markscheme solution below;

Mythoughts on this; (Alternative Method)
i used the simultaneous equation
$λ$$\left[\dfrac {1}{2}a -\dfrac {1}{4}b\right]$=$\left[ -\dfrac {3}{4}b+ ka\right]$ where $OR=k OA$
$- \dfrac {1}{4}bλ$=$-\dfrac {3}{4}b$ ⇒$λ=3$ and also
$\dfrac {3}{2}a = ka$ ⇒$k=1.5$ therefore $OA:OR=2:3$ ⇒$OA:AR=2:1$

your thoughts guys...

 

[anuttarasammyak]

Say middle point of OB is S, we know SA and PR are parallel. Similarity of triangles POR and SOA gives OA:AR=2:1.

 

[chwala]

Say middle point of OB is S, we know SA and PR are parallel. Similarity of triangles POR and SOA gives OA:AR=2:1.

Thanks Anutta...its always good to explore different ways in solving Math problems...by doing so i realize that i become better...cheers