- #1
anemone
Gold Member
MHB
POTW Director
- 3,883
- 115
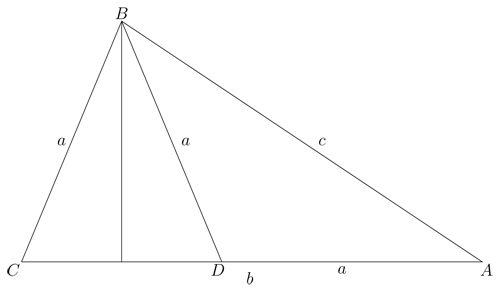
Let \(\displaystyle ABC\) be a triangle such that \(\displaystyle \frac{BC}{AB-BC}=\frac{AB+BC}{AC}\).
Determine the ratio \(\displaystyle \frac{\angle A}{\angle C}\).
Determine the ratio \(\displaystyle \frac{\angle A}{\angle C}\).