Determining experimental mass from graph

[tk95]

So this question is from a physics lab. The apparatus for the experiment was made upon a device that measures angular speed over time. On top of this is a rail to which different items can be attached. Directly in the center of the rail is a device which has a pulley with a string to attached it, and it has an indicator that goes up or down as the apparatus spins; we were told this measures centrifugal force. 16cm away from the center of the rail, is a hanging weight of unknown mass; this mass has hooks on it from which it can hang, attach to the super pulley, and also attach the last weight, which goes over the end of the rail over a pulley and hangs. We put different masses at the end, and then used the pulley device in the center to mark where the indicator hangs; then the mass is taken off, causing the indicator to move. Angular speed was then increased until the indicator moves back to where it was, and using this angular speed and the hanging mass (which was removed before the actual experiment) we are supposed to be able to determine the mass of the hanging object.

Sorry for the awful explanation - let me know if more clarification is needed.

EDIT: Here is an image of the experimental setup.

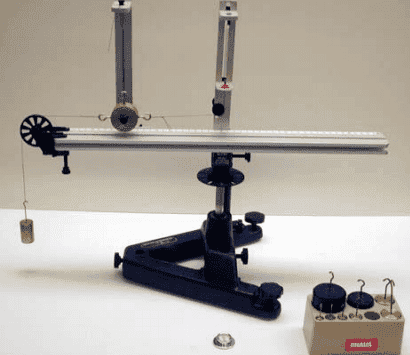
Anyways, once we had our data, we made a graph of Angular Speed squared (x-axis) and hanging mass (y-axis); this graph was linear and had an equation of y=3.26x+1.31. This graph was made for us based on our data, so even though those axes sound wrong (to me at least), that's how it's supposed to be. Anyways, using the equation for this graph, and the equation written below, we are supposed to be able to determine the experimental mass:

M = m * r / g * ω^2

where M is the experimental mass, m is the hanging mass, r is 16.00 cm, and ω/g obviously represent acceleration due to gravity and angular speed (squared).

I can not for the life of me figure out how to use these two equations to determine M.

Any help greatly appreciated!

 

[gneill]

Hi tk95, Welcome to Physics Forums.

In future, please use the template provided in the edit window to format your problem. This is in the forum rules.

Can you use parentheses in your expression to clearly spell out the order of operations? Is your equation:

a) M = m * (r/g) * ω²

b) M = m * r / (g * ω²)

I strongly suspect that it's (a).

Consider your equation to be of the form y = a x , where a represents the slope of a line. Can you find the sub-expression in your equation that represents the slope?

 

[tk95]

Hi tk95, Welcome to Physics Forums.

In future, please use the template provided in the edit window to format your problem. This is in the forum rules.

Can you use parentheses in your expression to clearly spell out the order of operations? Is your equation:

a) M = m * (r/g) * ω²

b) M = m * r / (g * ω²)

I strongly suspect that it's (a).

Consider your equation to be of the form y = a x , where a represents the slope of a line. Can you find the sub-expression in your equation that represents the slope?

Yes, it's (a).

I understand that the coefficient (3.26) represents the slope. The slope represents m / ω^2 (rise over run or y/x), which doesn't seem useful since this term is not in the other formula, m * (r/g) * ω²

 

[gneill]

Maybe there's a mixup in the mass naming? Can you derive the given formula using the physical principles involved in the experimental setup? The way I see it, according to the formula M should be the mass that's hanging off the end of the equipment and m the mass that gets spun around.

 

[tk95]

Maybe there's a mixup in the mass naming? Can you derive the given formula using the physical principles involved in the experimental setup? The way I see it, according to the formula M should be the mass that's hanging off the end of the equipment and m the mass that gets spun around.

This is from the lab:
You start with the magnitude of centripetal force acting on the object:
F net = m * v² /r

Tangential speed of an object in uniform motion:
v = 2 pi r / T (T is period of revolution)

Rotational speed: ω = 2 pi / T

so v = 2 * ω * r

Plug this back into initial equation,

F net = m (2 * ω * r)² / r

so

M * g = m * ω² * r

so

M = m * ω² * r / g

The only thing I'm putting in that isn't directly taken from the lab is that F net = M * g, maybe I'm wrong on this though. He stops the expression as F net = m * ω² * r

EDIT: For clarity, just see here: http://i.imgur.com/5Ctx821.png

 

[gneill]

Okay. So M is the dangling mass, and m the spinning (unknown) mass as I thought. Now you should be able to put your equation in the form of a linear (line) equation, and identify the part that represents the slope.

 

[tk95]

Okay. So M is the dangling mass, and m the spinning (unknown) mass as I thought. Now you should be able to put your equation in the form of a linear (line) equation, and identify the part that represents the slope.

Ok, so we rewrite as:

m = M * g / (r * ω²)

or we can also write as

m = (M / ω²) * (g / r)

since as I identified previously, the coefficient 3.26 represents M / ω². Plugging this in we get 3.26 * 9.8 / 16, which gives a value of 2.0 g. The hanging mass was definitely greater than 2 grams.

 

[gneill]

Your plot was of M vs ω². So automatically those become associated with your "y" and "x" variables. That is,

M == y and ω² == x.

What's left must be associated with the slope. So you have M = (?) ω². Fill in the "?".

 

[tk95]

Your plot was of M vs ω². So automatically those become associated with your "y" and "x" variables. That is,

M == y and ω² == x.

What's left must be associated with the slope. So you have M = (?) ω². Fill in the "?".

M = m * r / g * ω² becomes
y = m * r / g * x

so m * r / g = 3.26

Solving for m, you get m = 3.26 * g / r. This is the same as in my previous reply, 1.997 or about 2. Am I still missing something here?

 

[gneill]

Solving for m, you get m = 3.26 * g / r. This is the same as in my previous reply, 1.997 or about 2. Am I still missing something here?

That depends. What units were associated with the values? Make sure that everything is consistent. The slope will have units associated with it.

 

[tk95]

That depends. What units were associated with the values? Make sure that everything is consistent. The slope will have units associated with it.

You were right, it's because of the units. The radius was measured in centimeters but the standard units for g are in meters, so the real answer is 3.26 grams/(rad²/s²) * 9.80 m/s² * 100 cm / 1m / 16.00cm = 199.7 grams. Does that sound right?

 

[gneill]

You were right, it's because of the units. The radius was measured in centimeters but the standard units for g are in meters, so the real answer is 3.26 grams/(rad²/s²) * 9.80 m/s² * 100 cm / 1m / 16.00cm = 199.7 grams. Does that sound right?

Looks like a reasonable value to me, given the apparent size of the mass in the image.