kodama
- 1,075
- 144
- TL;DR Summary
- Gravitational self-interaction cannot replace dark matter
Deur Gravitational self-interaction Doesn't Explain Galaxy Rotation Curves
this paper
A. N. Lasenby, M. P. Hobson, W. E. V. Barker, "Gravitomagnetism and galaxy rotation curves: a cautionary tale" arXiv:2303.06115 (March 10, 2023).
Directly comments on Deur's theory of self-interaction

 the question is why if Deur is correct why has his results been missed by numerical general relativity and other approximations by highly qualified GR experts
the question is why if Deur is correct why has his results been missed by numerical general relativity and other approximations by highly qualified GR experts
 these GR experts found NO support for Deur's claims, including use of super computers.
these GR experts found NO support for Deur's claims, including use of super computers.
authors state

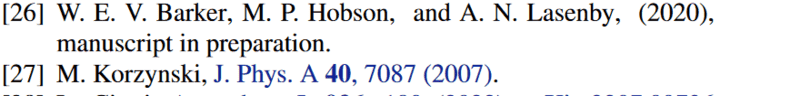
Mikołaj Korzyński1 Published 6 June 2007 • 2007 IOP Publishing Ltd
Journal of Physics A: Mathematical and Theoretical, Volume 40, Number 25 Citation Mikołaj Korzyński 2007 J. Phys. A: Math. Theor. 40 7087 DOI 10.1088/1751-8113/40/25/S66
Cooperstock and Tieu proposed a model of galaxy, based on ordinary GR, in which no exotic dark matter is needed to explain the flat rotation curves in galaxies. I will present the arguments against this model. In particular, I will show that in their model the gravitational field is generated not only by the ordinary matter distribution, but by a infinitely thin, massive and rotating disc as well. This is a serious and incurable flaw and makes the Cooperstock–Tieu metric unphysical as a galaxy model.So Deur's claims Gravitational self-interaction can replace dark matter with just ordinary GR + ideas from QCD that are non-viable, the authors go on to show that GEM also does not work as too weak by a factor of 10-6neither Gravitational self-interaction per Deur nor GEM and standard GR can replace dark matter (or MOND)
time to move on. perhaps refracted gravity is a better approach
Valentina Cesare
this paper
A. N. Lasenby, M. P. Hobson, W. E. V. Barker, "Gravitomagnetism and galaxy rotation curves: a cautionary tale" arXiv:2303.06115 (March 10, 2023).
We investigate recent claims that gravitomagnetic effects in linearised general relativity can explain flat and rising rotation curves, such as those observed in galaxies, without the need for dark matter.
If one models a galaxy as an axisymmetric, stationary, rotating, non-relativistic and pressureless 'dust' of stars in the gravitoelectromagnetic (GEM) formalism, we show that GEM effects on the circular velocity v of a star are O(10^−6) smaller than the standard Newtonian (gravitoelectric) effects.
Moreover, we find that gravitomagnetic effects are O(10^−6) too small to provide the vertical support necessary to maintain the dynamical equilibrium assumed.
These issues are obscured if one constructs a single equation for v, as considered previously. We nevertheless solve this equation for a galaxy having a Miyamoto--Nagai density profile. We show that for the values of the mass, M, and semi-major and semi-minor axes, a and b, typical for a dwarf galaxy, the rotation curve depends only very weakly on M. Moreover, for aspect ratios a/b>2, the rotation curves are concave over their entire range, which does not match observations in any galaxy.
Most importantly, we show that for the poloidal gravitomagnetic flux ψ to provide the necessary vertical support, it must become singular at the origin. This originates from the unwitting, but forbidden, inclusion of free-space solutions of the Poisson-like equation that determines ψ, hence ruling out the methodology as a means of explaining flat galaxy rotation curves.
We further show that recent deliberate attempts to leverage such free-space solutions against the rotation curve problem yield no deterministic modification outside the thin disk approximation, and that, in any case, the homogeneous contributions to ψ are ruled out by the boundary value problem posed by any physical axisymmetric galaxy.
Directly comments on Deur's theory of self-interaction
authors state
Can dark matter in galaxies be explained by relativistic corrections?
Mikołaj Korzyński1 Published 6 June 2007 • 2007 IOP Publishing Ltd
Journal of Physics A: Mathematical and Theoretical, Volume 40, Number 25 Citation Mikołaj Korzyński 2007 J. Phys. A: Math. Theor. 40 7087 DOI 10.1088/1751-8113/40/25/S66
Abstract
Cooperstock and Tieu proposed a model of galaxy, based on ordinary GR, in which no exotic dark matter is needed to explain the flat rotation curves in galaxies. I will present the arguments against this model. In particular, I will show that in their model the gravitational field is generated not only by the ordinary matter distribution, but by a infinitely thin, massive and rotating disc as well. This is a serious and incurable flaw and makes the Cooperstock–Tieu metric unphysical as a galaxy model.So Deur's claims Gravitational self-interaction can replace dark matter with just ordinary GR + ideas from QCD that are non-viable, the authors go on to show that GEM also does not work as too weak by a factor of 10-6neither Gravitational self-interaction per Deur nor GEM and standard GR can replace dark matter (or MOND)
time to move on. perhaps refracted gravity is a better approach
Dark Coincidences: Small-Scale Solutions with Refracted Gravity and MOND
Valentina Cesare
General relativity and its Newtonian weak field limit are not sufficient to explain the observed phenomenology in the Universe, from the formation of large-scale structures to the dynamics of galaxies, with the only presence of baryonic matter. The most investigated cosmological model, the ΛCDM, accounts for the majority of observations by introducing two dark components, dark energy and dark matter, which represent ∼95% of the mass-energy budget of the Universe. Nevertheless, the ΛCDM model faces important challenges on the scale of galaxies. For example, some very tight relations between the properties of dark and baryonic matters in disk galaxies, such as the baryonic Tully-Fisher relation (BTFR), the mass discrepancy-acceleration relation (MDAR), and the radial acceleration relation (RAR), which see the emergence of the acceleration scale a0≃1.2×10−10 m s−2, cannot be intuitively explained by the CDM paradigm, where cosmic structures form through a stochastic merging process. An even more outstanding coincidence is due to the fact that the acceleration scale a0, emerging from galaxy dynamics, also seems to be related to the cosmological constant Λ. Another challenge is provided by dwarf galaxies, which are darker than what is expected in their innermost regions. These pieces of evidence can be more naturally explained, or sometimes even predicted, by modified theories of gravity, that do not introduce any dark fluid. I illustrate possible solutions to these problems with the modified theory of gravity MOND, which departs from Newtonian gravity for accelerations smaller than a0, and with Refracted Gravity, a novel classical theory of gravity introduced in 2016, where the modification of the law of gravity is instead regulated by a density scale.
| Comments: | 34 pages, 7 figures, published on 16th January 2023 in Universe 2023, 9(1), 56, in the Special Issue "Modified Gravity and Dark Matter at the Scale of Galaxies"; accepted for publication on 12th January 2023 |
| Subjects: | Astrophysics of Galaxies (astro-ph.GA) |
| Cite as: | arXiv:2301.07115 [astro-ph.GA] |

