Diameter of nanoparticles formed by dewetting of thin film

[Nick89]

Hi,

I'm trying to understand the derivation to an equation I found in the following paper: http://pubs.acs.org/doi/abs/10.1021/nn203035x

I posted almost this exact question on Physics Stack Exchange but got no replies so I decided to try it here instead, I hope that's ok!
http://physics.stackexchange.com/questions/306409/diameter-of-nanoparticles-formed-by-solid-state-dewetting-of-thin-filmsThe paper discusses the solid state dewetting of an ultrathin (~nanometer thickness) metal film into small nanoparticles. Specifically they state that the nanoparticle diameter $D$ will depend on the initial film thickness $h$ and the contact angle with the surface $\theta$ as:

$$D = \frac{6h\,\sin \theta}{1-\cos\theta}$$
for $\theta<90^{\circ}$ or
$$D = \frac{6h}{1-\cos\theta}$$
for $\theta>90^{\circ}$

First of all I don't understand how they arrive at this equation. Secondly I am not sure what they define as the 'particle diameter' $D$.

As far as I can tell the only other equations they use are the interfacial energies and Young's equation which gives the contact angle:

The interfacial energy of an ideal thin film of thickness $h$ on a substrate is given by:
$$G_1 = A(\sigma_1+\sigma_{12})$$
where $\sigma_1$ is the catalyst surface energy, $\sigma_{12}$ is the catalyst-support interface energy and $A$ is the support area per nanoparticle.

Question 1: what is $A$ exactly? They're talking about a thin film of thickness $h$ but at the same time the equation includes the "support area per nanoparticle"??

Next, the interfacial energy of a truncated spherical ball of top surface area $S_2$ in contact with the flat surface is given by:
$$G_2 = (A - S_{12})\sigma_2 + S_2\sigma_1 + S_{12}\sigma_{12}$$
where $\sigma_2$ is the support surface energy and $S_{12}$ is the contact area.

Question 2: What is the "contact area" $S_{12}$ exactly and how is it different than the "support area per nanoparticle" $A$??

Also, we have Young's equation:
$$\sigma_2= \sigma_{12}+\sigma_1 \cos\theta$$

Finally, the paper states that the minimum diameter of the nanoparticles occurs when $G_2 = G_1$. Just setting $G_2 = G_1$ one easily arrives at this relation which is probably useful:
$$A = S_2 - S_{12}\cos\theta$$

Now I am stuck however. I don't know the difference between $A$ and $S_{12}$ and maybe even $S_2$ (but I assume $S_2$ is the surface area of the spherical cap). The only other argument they use is "conservation of catalyst volume", e.g. no material is lost. Without further explanation they state the equations for $D$ that I mentioned at the top.

Question 3: How can we derive the equation for $D$ from these relations?

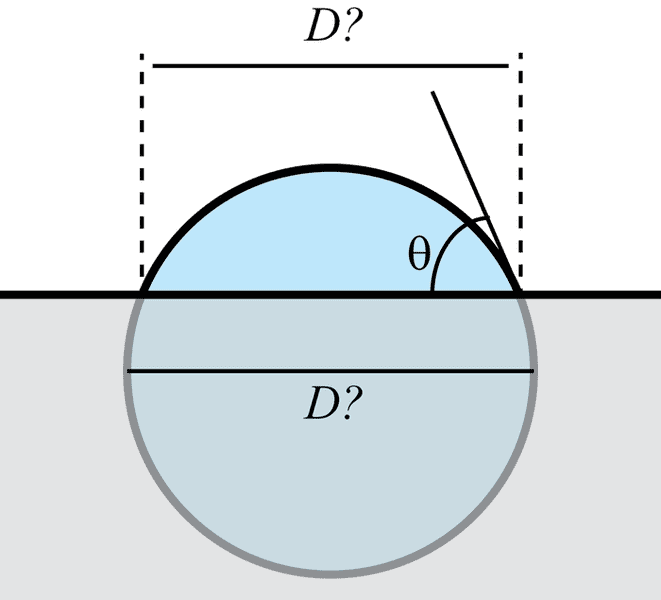
Question 4: And one final question that might be important: how do we define the diameter $D$ if the contact angle $\theta$ is below 90 degrees? See the picture at the bottom. Is $D$ the diameter of the particle that we would observe if we looked from the top, e.g. the "base"? Or is it the diameter of the particle if we imagine it extending below the surface?I must be missing a crucial step because I can't figure out how to get to the equations for $D$ above. I figured some math on spherical caps may be useful so I found this:
http://mathworld.wolfram.com/SphericalCap.html

This page uses the height of the particle which I don't know, so I derived an equation for the volume of the particle as a function of only the contact angle $\theta$ and the "observed radius" (which the above page calls the base $a = d/2$):
$$V = \frac{\pi d^3}{48} \tan\frac{\theta}{2} \left(3 + \tan^2\frac{\theta}{2} \right)$$

Similarly for the surface area of the cap:
$$S = \frac{\pi d^2}{4}\left(1 + \tan^2\frac{\theta}{2}\right)$$

Pretty sure these are only valid for $\theta<90$ though.

Not sure if these are helpful...

I am trying to apply this equation to my research but it's not even close, so I would like to know where it comes from to decide if it's valid or not and how I should treat my results...

Thanks for any help!

 

[Nick89]

Nobody? :(

I have gotten a little bit further I believe. I imagined the thin film being cut up into many square blocks, where each block is transformed into one particle. Therefore the volume of such a block should be equal to the volume of the particle. I called the width and length of the blocks $w$ so its volume is $hw^2$ (as $h$ is the initial film thickness).

I made the following assumptions:

• $A$ is the surface area of such an initial block. Therefore $A = w^2$.
• $S_2$ is the surface area of the spherical cap, which is $S_2 = \frac{\pi d^2}{4} \left(1+\tan\frac{\theta}{2}\right)$
• $S_{12}$ is the bottom / contact surface of the particle: $S_{12} = \frac{\pi d^2}{4}$

Then using $A = w^2 = S_2 - S_{12}\cos\theta$ I have an expression for $w$.

Finally,
$$V_{\rm{block}} = V_{\rm{particle}}$$
$$hw^2 = h \left(S_2 - S_{12}\cos\theta \right) = V_{\rm{particle}} = \frac{\pi d^3}{48} \tan \frac{\theta}{2} \left(3 + \tan^2 \frac{\theta}{2}\right)$$

When I solve this equation for $d$ using Mathematica and simplify the result, it tells me (remarkably) that the solution is
$$d = 6h\sin\theta$$

Close... But not quite what the paper states, I'm missing the $1-\cos\theta$ fraction for some reason.

I can't see where I'm going wrong in my math. Maybe my definition of the diameter is not the same after all?