guyvsdcsniper
- 264
- 37
- Homework Statement
- What expressions give the magnetic field at the point r due to the moving charge?
- Relevant Equations
- Biot-Savart Law
My book never references the Biot-Savart law with in terms of 1/r^3, only 1/r^2.
How is it that it can be expressed as 1/r^3 as well
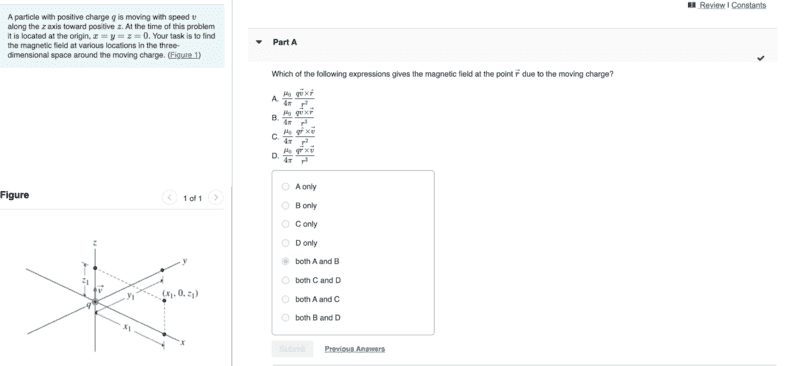
How is it that it can be expressed as 1/r^3 as well