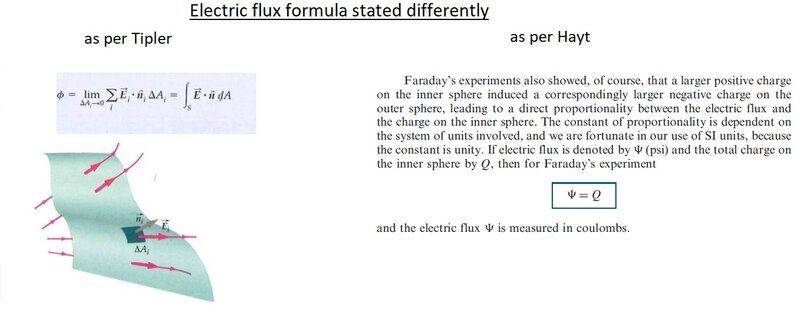
The discussion highlights discrepancies in electric flux formulas between different textbooks, specifically Tipler and Hayt. Tipler defines electric flux through a surface using the electric field (E), while Hayt presents it in terms of electric flux density (D) over an enclosed surface. Participants note the importance of distinguishing between electric flux and electric flux density, suggesting that the term "displacement flux density" might be more appropriate for D. The conversation also emphasizes that while the definitions differ, they can be reconciled through unit consistency, as both ultimately relate to charge (Q). Overall, the complexities of these definitions reflect deeper principles in electromagnetism and the importance of clarity in terminology.