Different ways of solving a problem

[Yankel]

Hello all,

I have an unusual question to the mathematicians here, and I hope that this is the right forum (if not, please move it to the appropriate board).

I am trying to come up with a mathematical task, from the Algebra field (middle school - early high school level), which can be solved in various ways (at least 5 various ways).

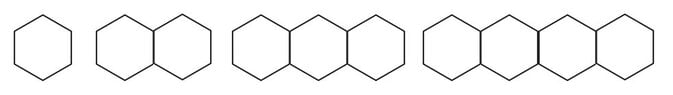
I will give you an example. In the attached photo, you see hexagons. The task is to find the perimeter in each stage (one hexagon, two hexagons, etc... up to many hexagons) assuming that the length of an edge is 1. This gives an arithmetic series.

One possible solution is to count how many edges are in the perimeter, to get a series (6, 10, 14, etc...), and from the series to realize the solution is 4n+2 where n is the number of hexagons. Another way of solving is to count the upper edges, to multiply by 2 and to add 2, it also gives the same answer. A third way can be multiplying the number of hexagons by 6 and reducing the common edges twice. A fourth way, although not suitable for middle school, is linear regression.

I need to come up with a similar idea, anything in middle school algebra (including functions, series, , probability and statistics - anything but geometry), which students can approach in at least 5 different ways and get to the same answer.

I am thinking about this for several days, but have no ideas. Can you please assist me with creative ideas ? The purpose of this assignment is educational, to give this task to kids to work on together, where they will come up with different ideas and learn from each other.

Any help is mostly appreciated !

View attachment 6175

 

[Kiwi1]

How about this problem: add all of the numbers 1,2,3,...,1000.

You could take it at face value and add them sequentially

You could re-order them to get 1000+(1+999) + (2+998) + ... and sum them up that way

You could re-order them in the same way but recognise how the series will terminate
to get 1000+(1+999) + (2+998) + ... +(499+501)+500 = 1000 + 499*1000+500

You could re-order them in another way
to get (1+1000)+(2+999) + (3+998) + ... +(500+501) = 500*1001

You could generalise the problem to n and find an explicit equation:
\(sum=\frac{n(n+1)}2\)

 

[Yankel]

Thank you Kiwi, that's a very good idea ! Definitely will be considered among the top ideas. If you have other ideas for to choose from, it will be great. The only problem with your idea, is that for some students it may be slightly too hard, but again, I find it good and will consider using it.

I had an idea of solving a system of two equations. However, I wish to get 5 different solutions, and I so far found only 3 for solving a system: Isolating one variable, subtracting one equation from another after multiplying, and graphically (we assume that in middle school matrices and Cramer rule are out of the question).

 

[HallsofIvy]

With edge length 1, each hexagon has circumference 6 so 6 hexagons have total circumference 6n. But you are not counting the sides between two hexagons. With n hexagons, there are n-1 line between them and those each count for two hexagons. So the total perimeter for n hexagons, connected like that, is 6n- 2(n-1)= 4n+ 2.

another way of looking at it: for the "internal hexagons" there are 4 sides because we are not counting the two attached the connected hexagons. For the two end hexagons, there are 5= 4+1 sides because we have to count the "end" sides. So there are 4 for every hexagon plus the 2 ends: 4n+ 2.

As a check, for 1 hexagon that is 4+ 2= 6, for 2 hexagons, 8+ 2= 10, for 3, 12+ 2= 14, for 4, 16+ 2= 18. Compare those to your examples shown.

 

[Yankel]

HallsofIvy,

Thank you for the nice explanation. The hexagons problem is solved (was solved before I posted it), I am looking for a "similar" problem.