Lhh
- 3
- 0
I’m struggling with questions c, e and f.
I don’t think I understand how to find stationary points.
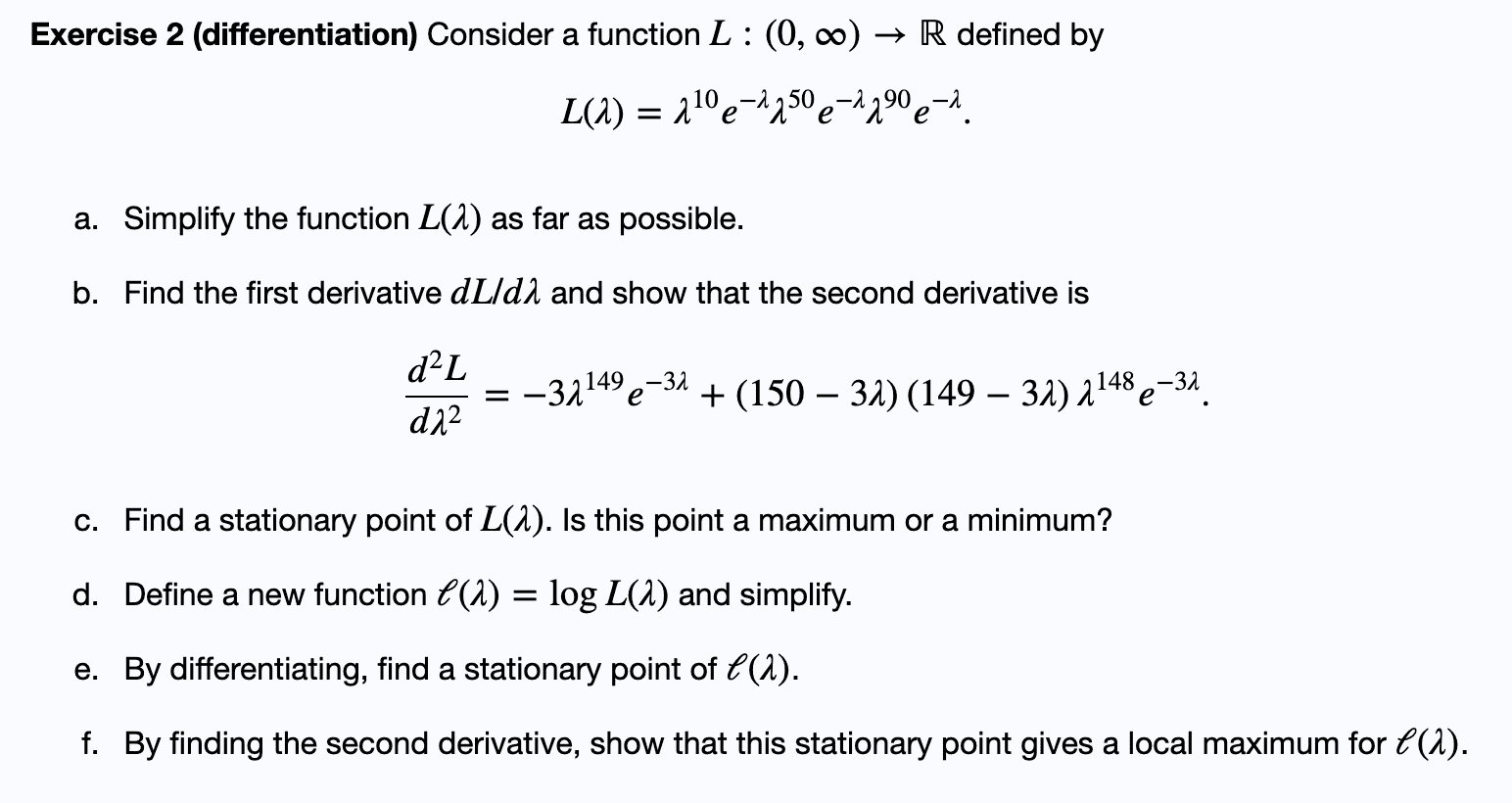
I don’t think I understand how to find stationary points.
Last edited by a moderator: