- #1
PhiowPhi
- 203
- 8
In the following diagrams:
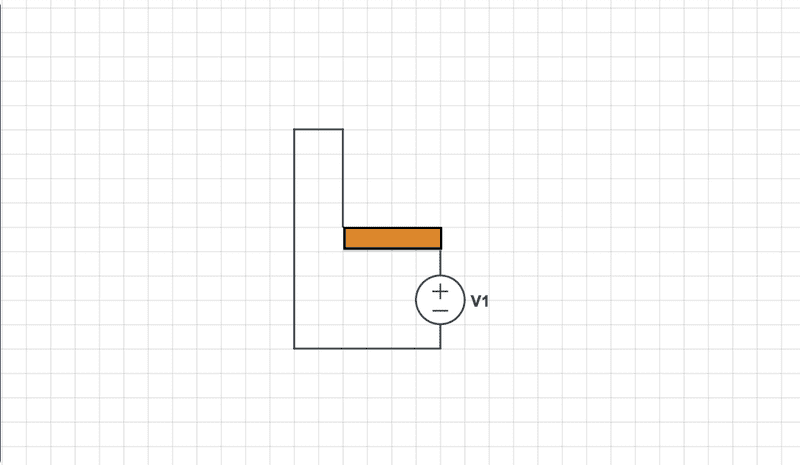
and:
Is current flowing horizontal or vertical&horizontal?
I'm sure for the rectangle one the flow of current is horizontal, but not sure of that square.
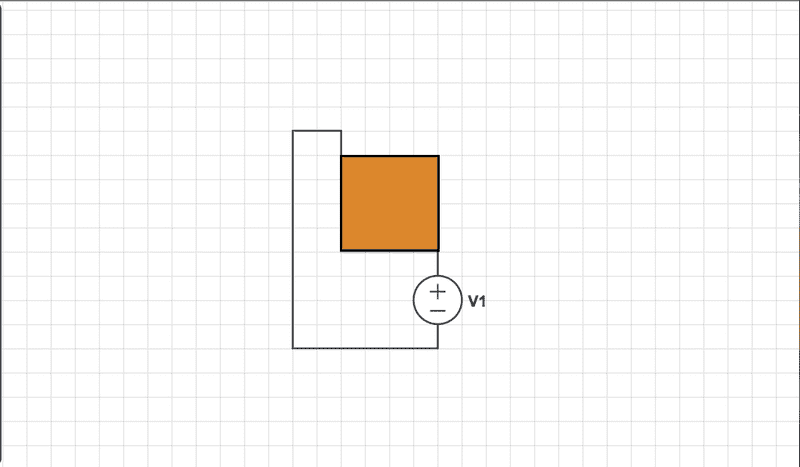
and:
Is current flowing horizontal or vertical&horizontal?
I'm sure for the rectangle one the flow of current is horizontal, but not sure of that square.